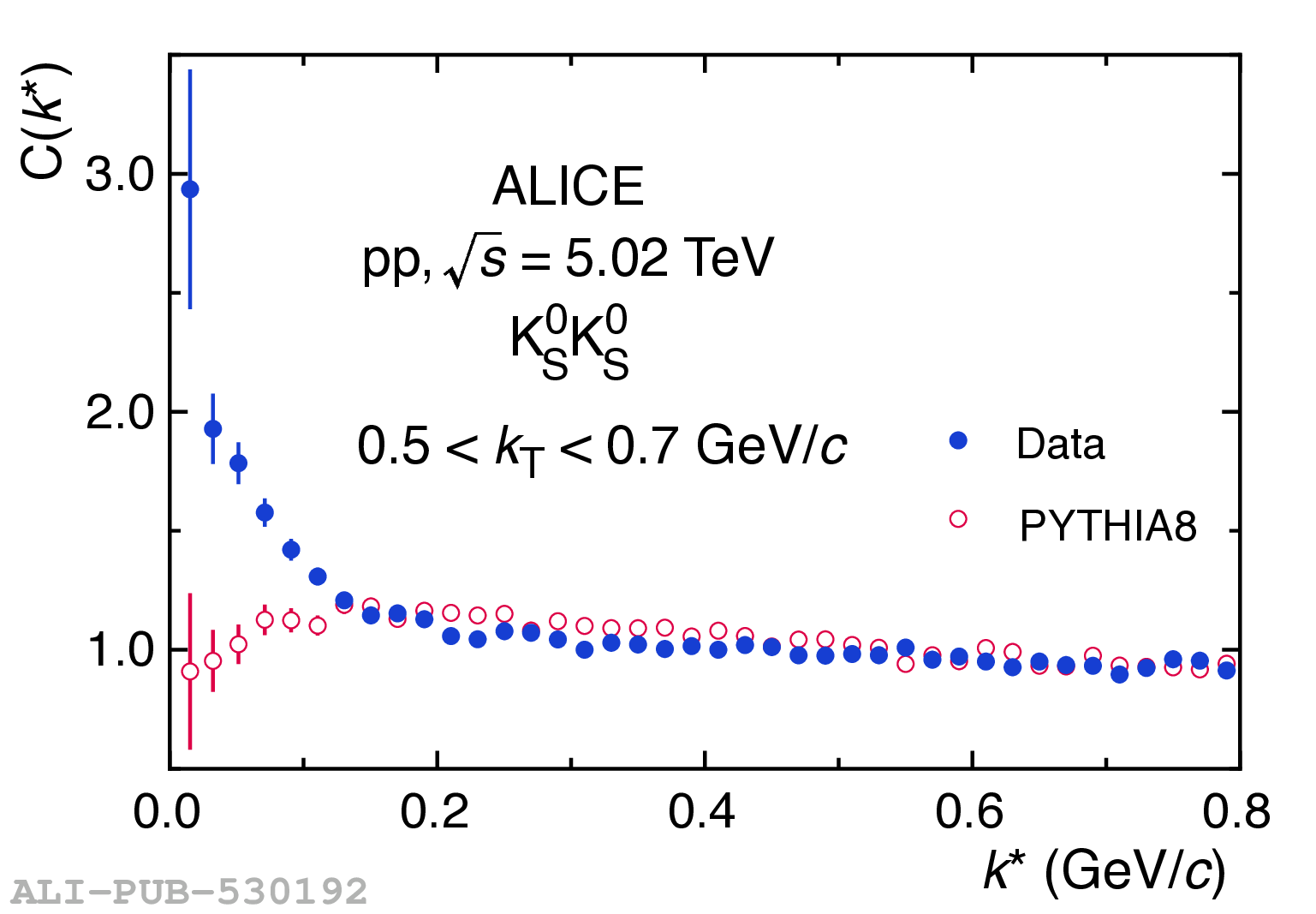
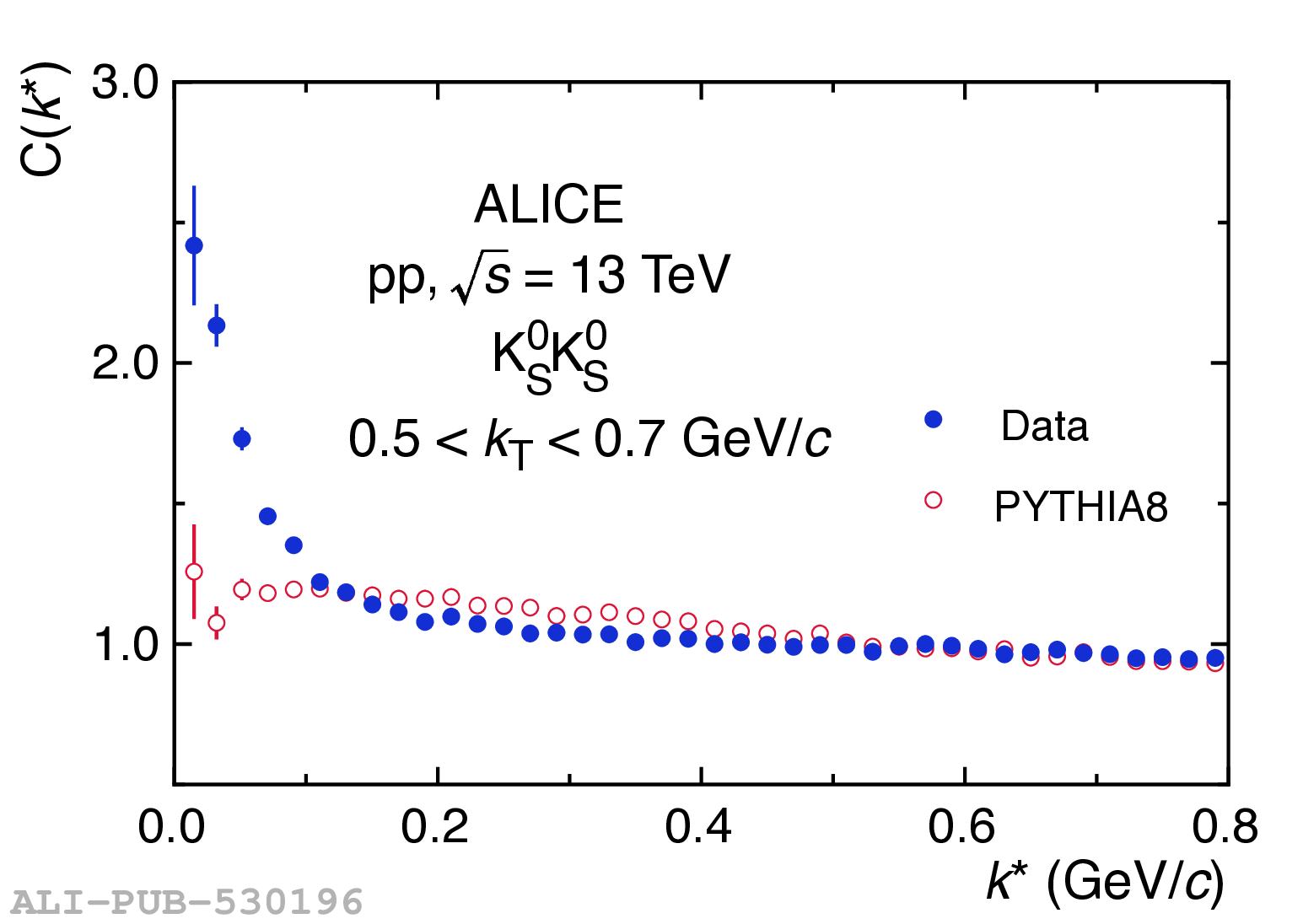
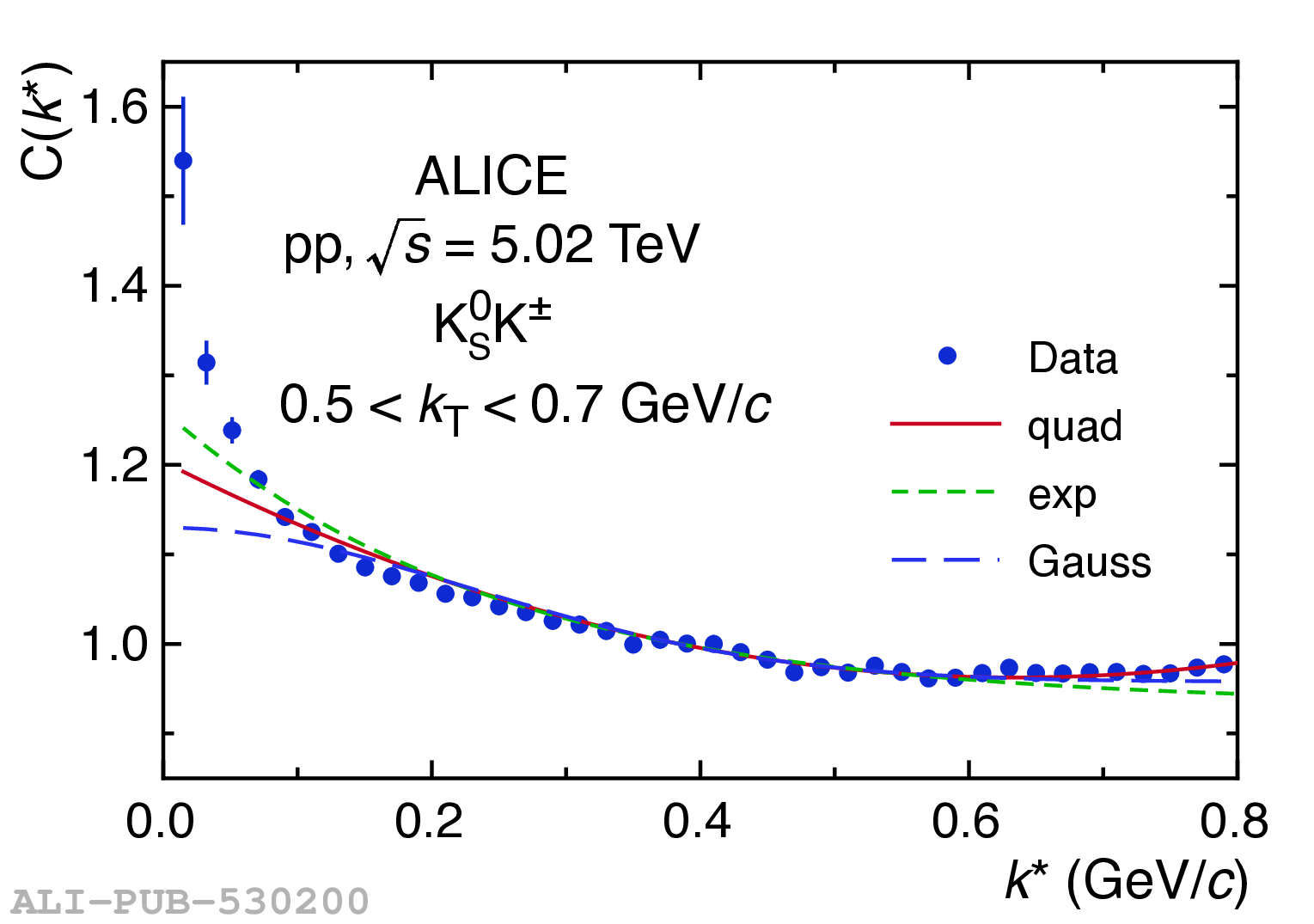
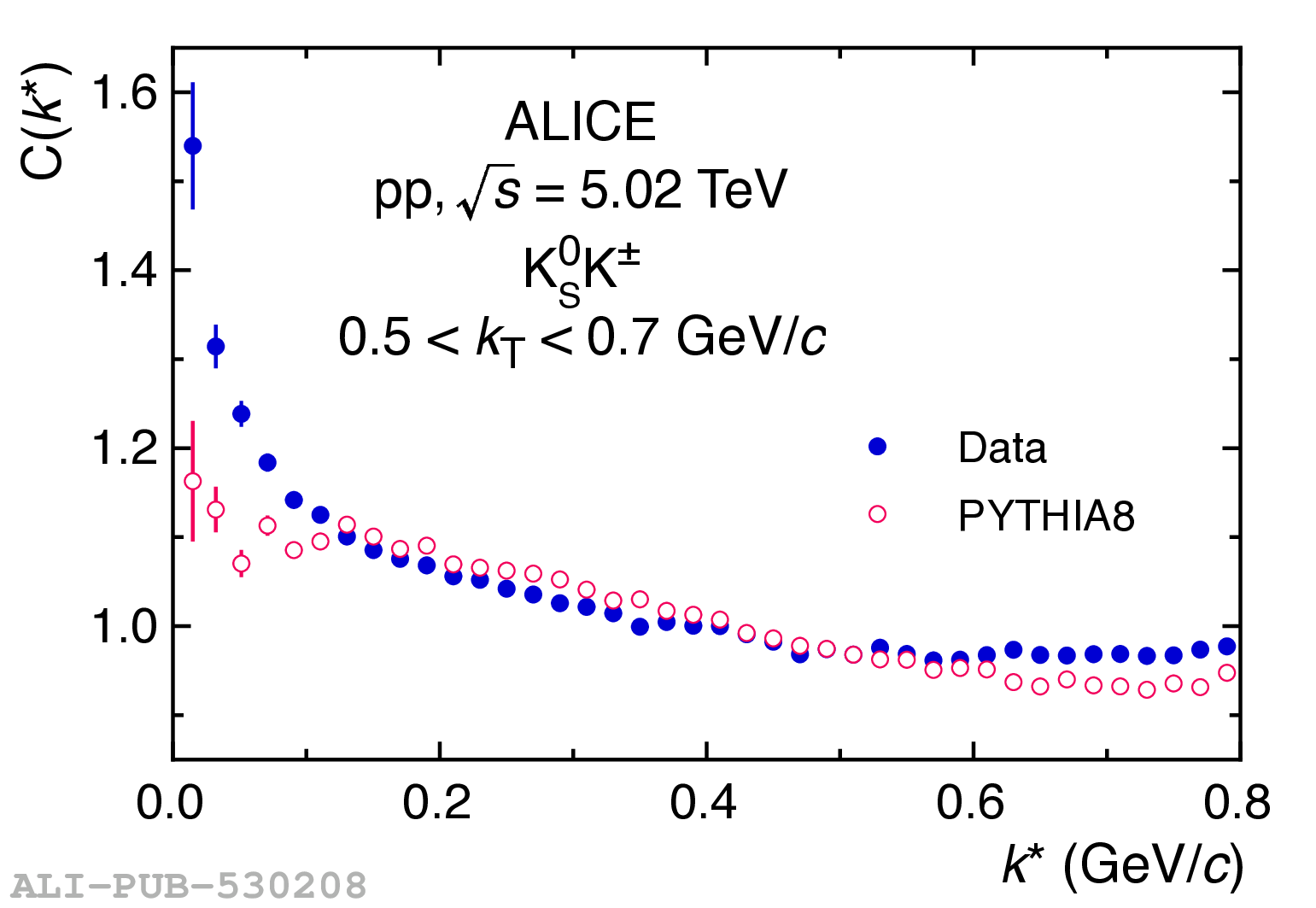
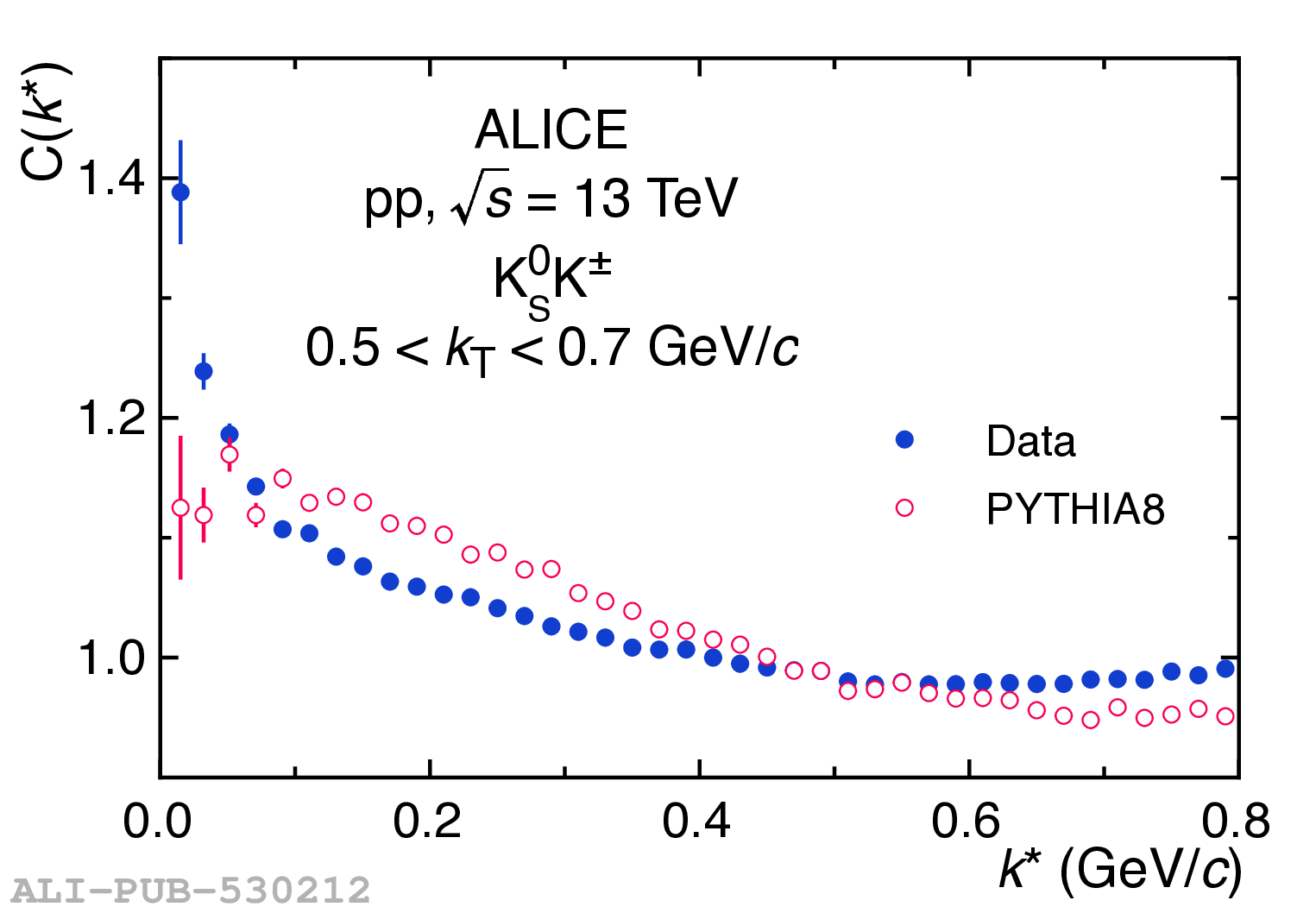
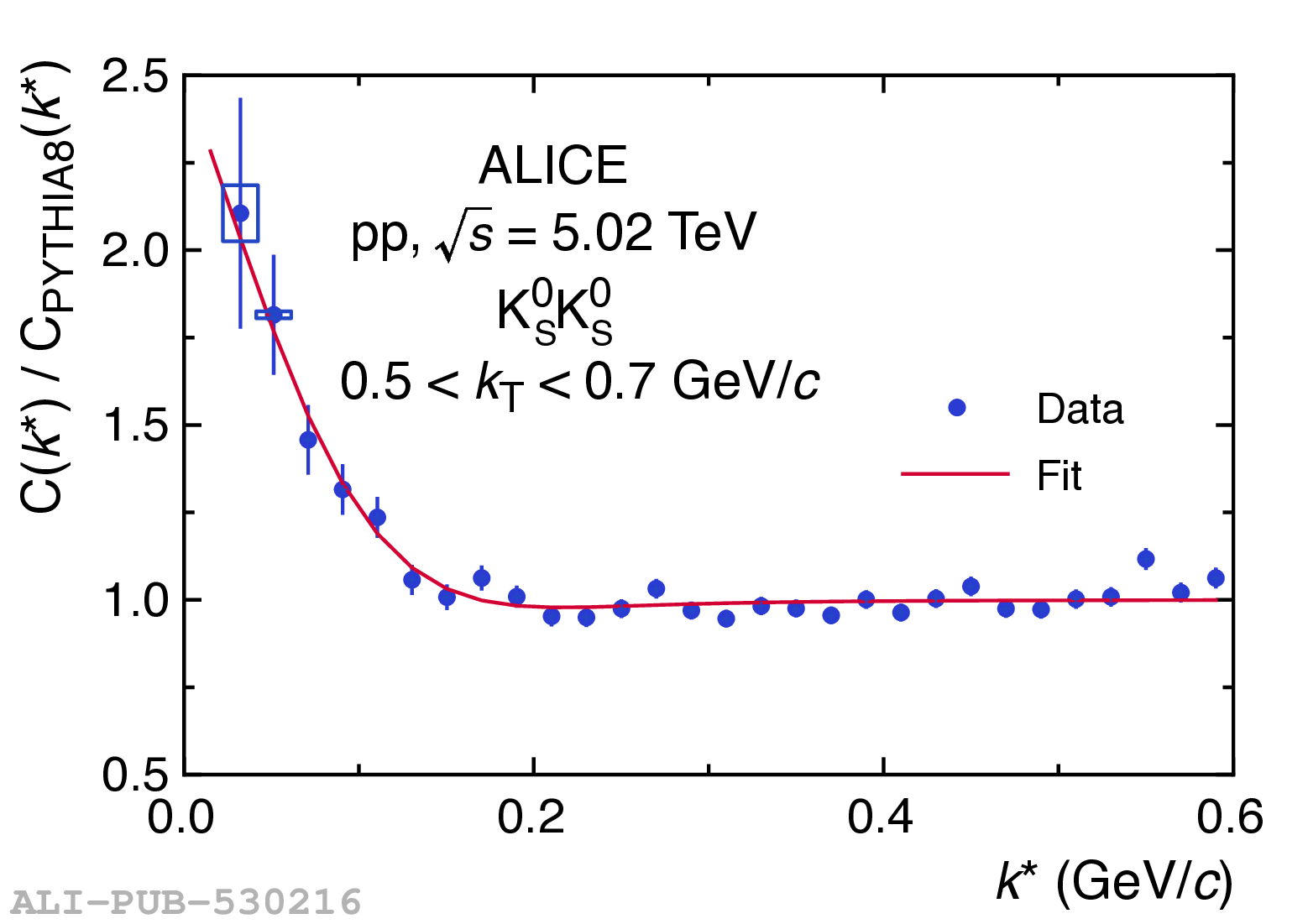
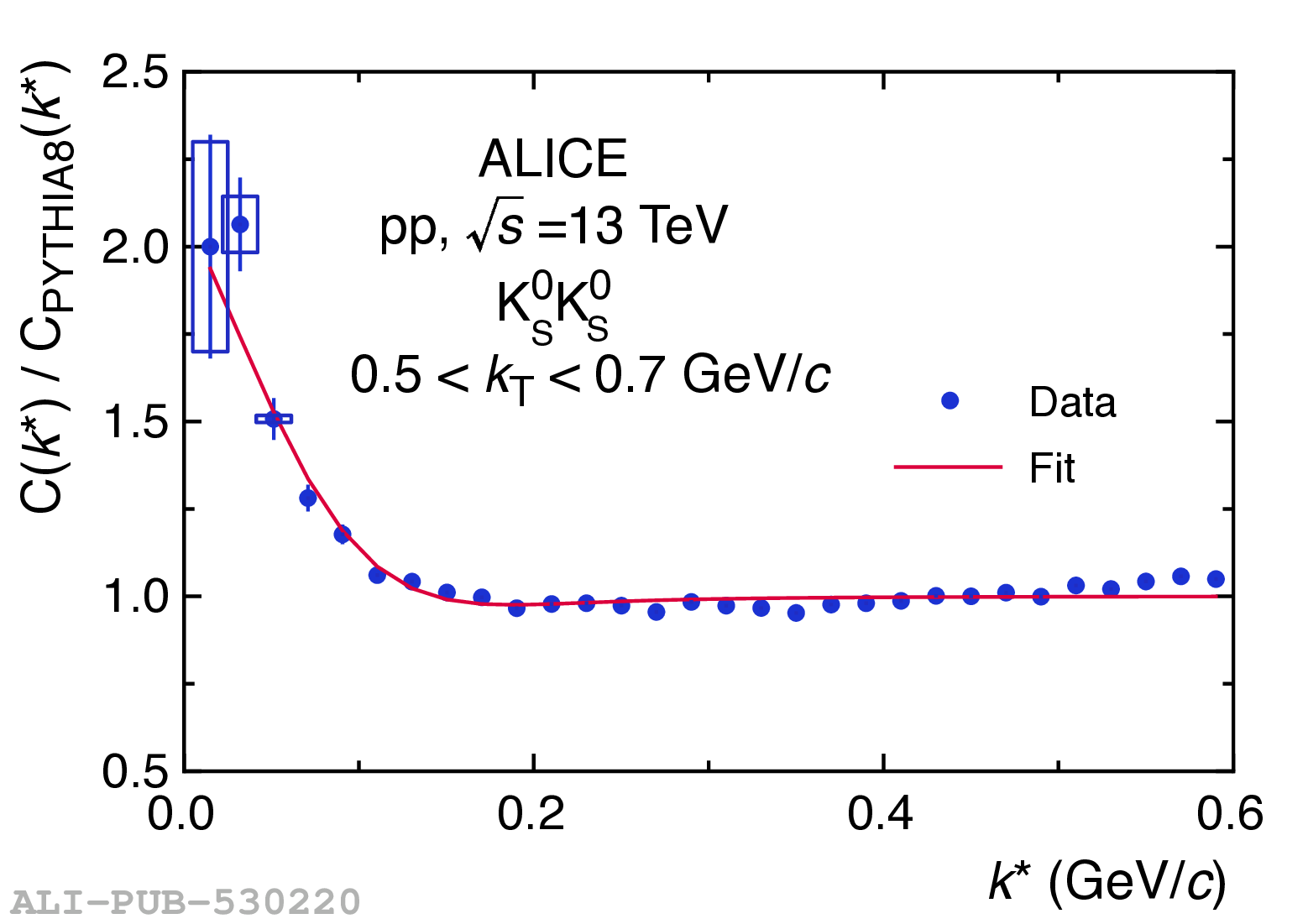
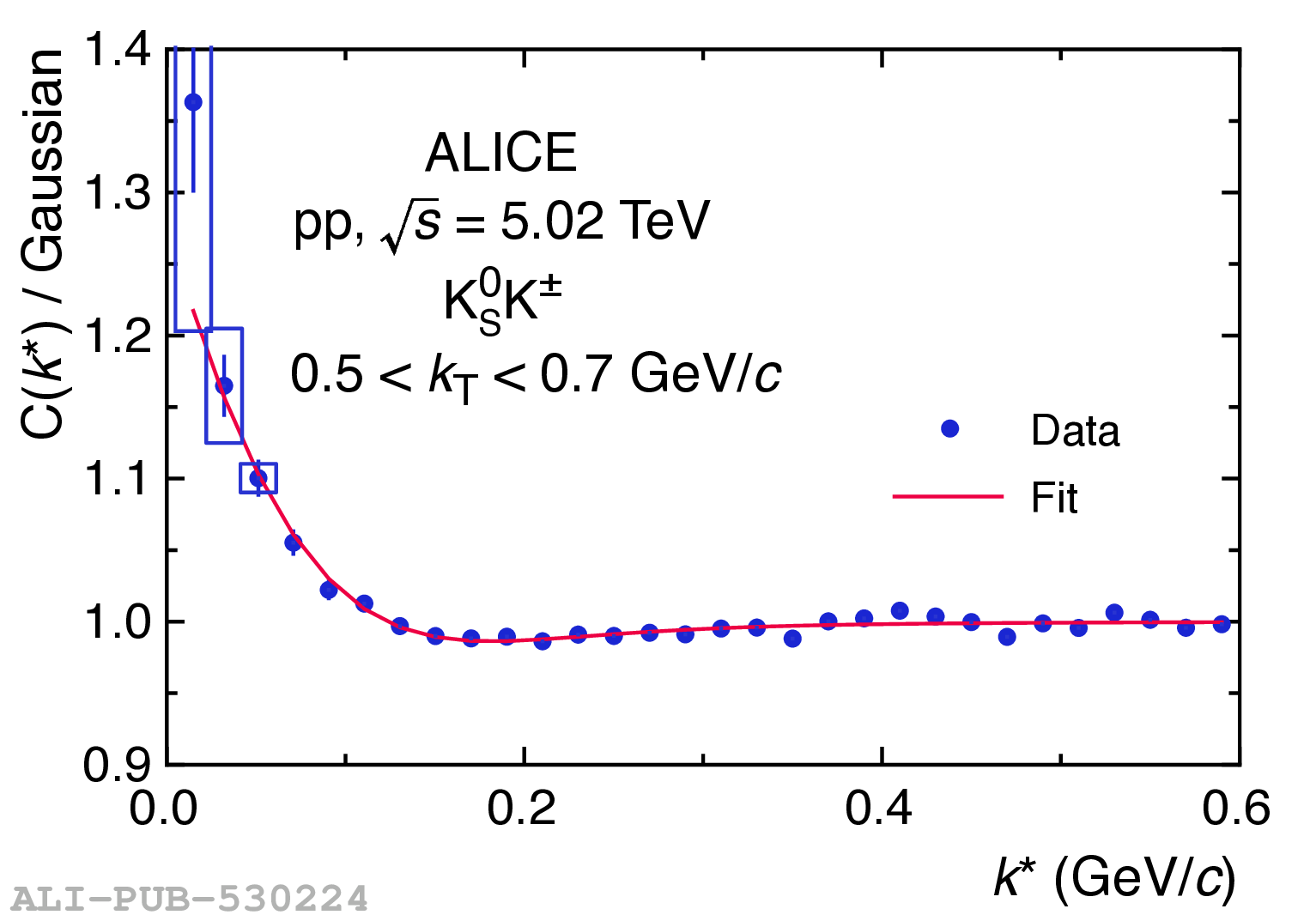
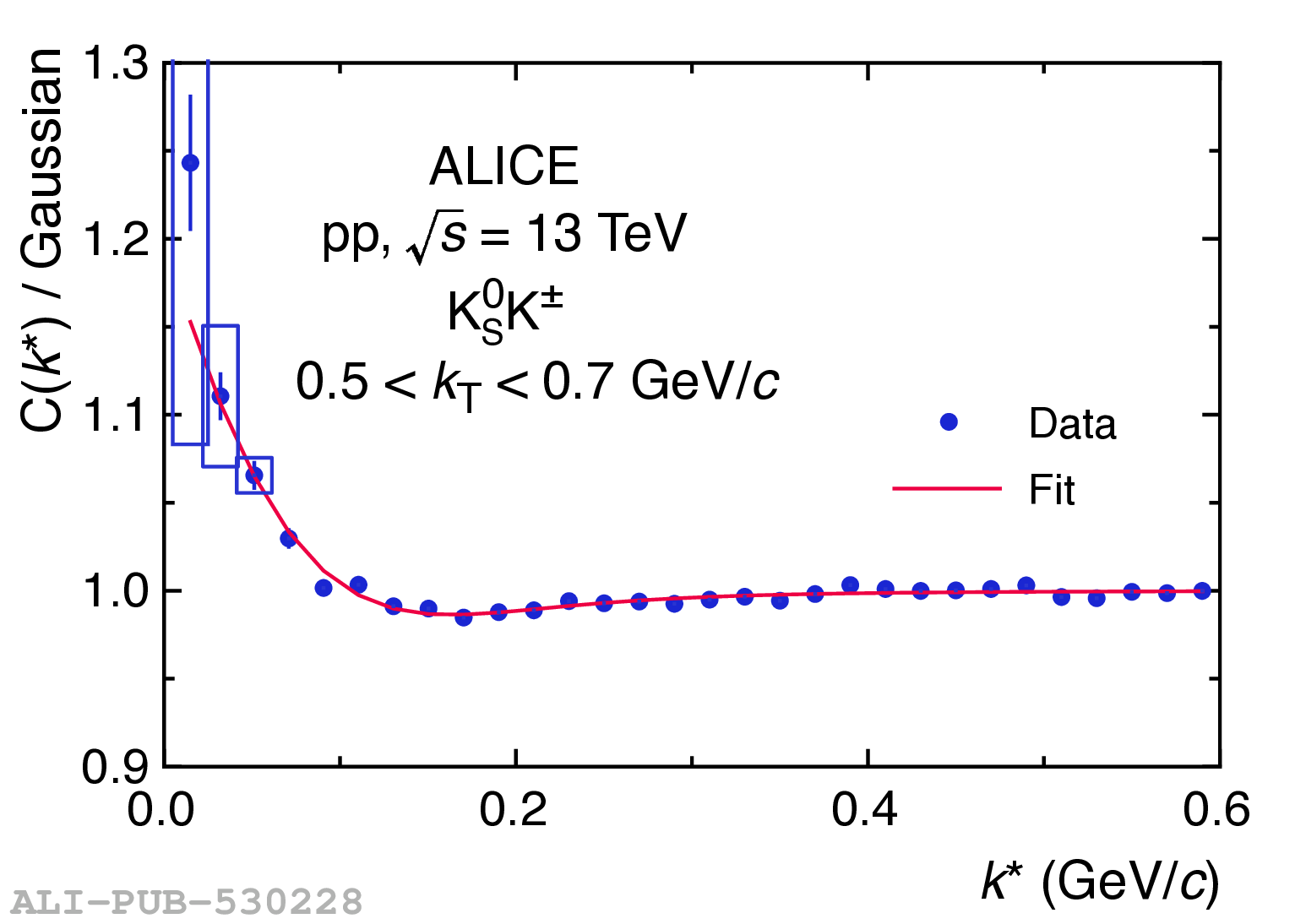
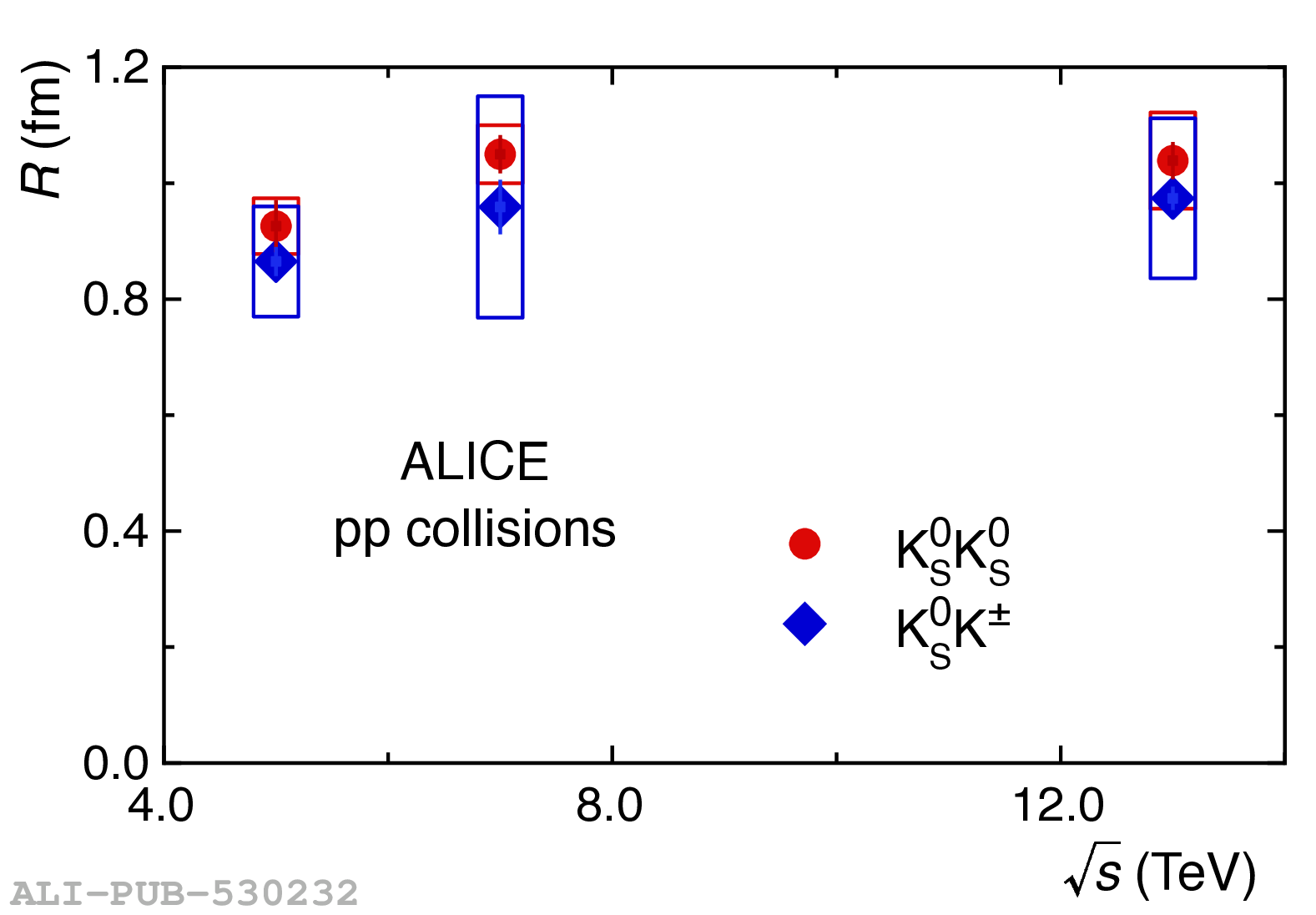
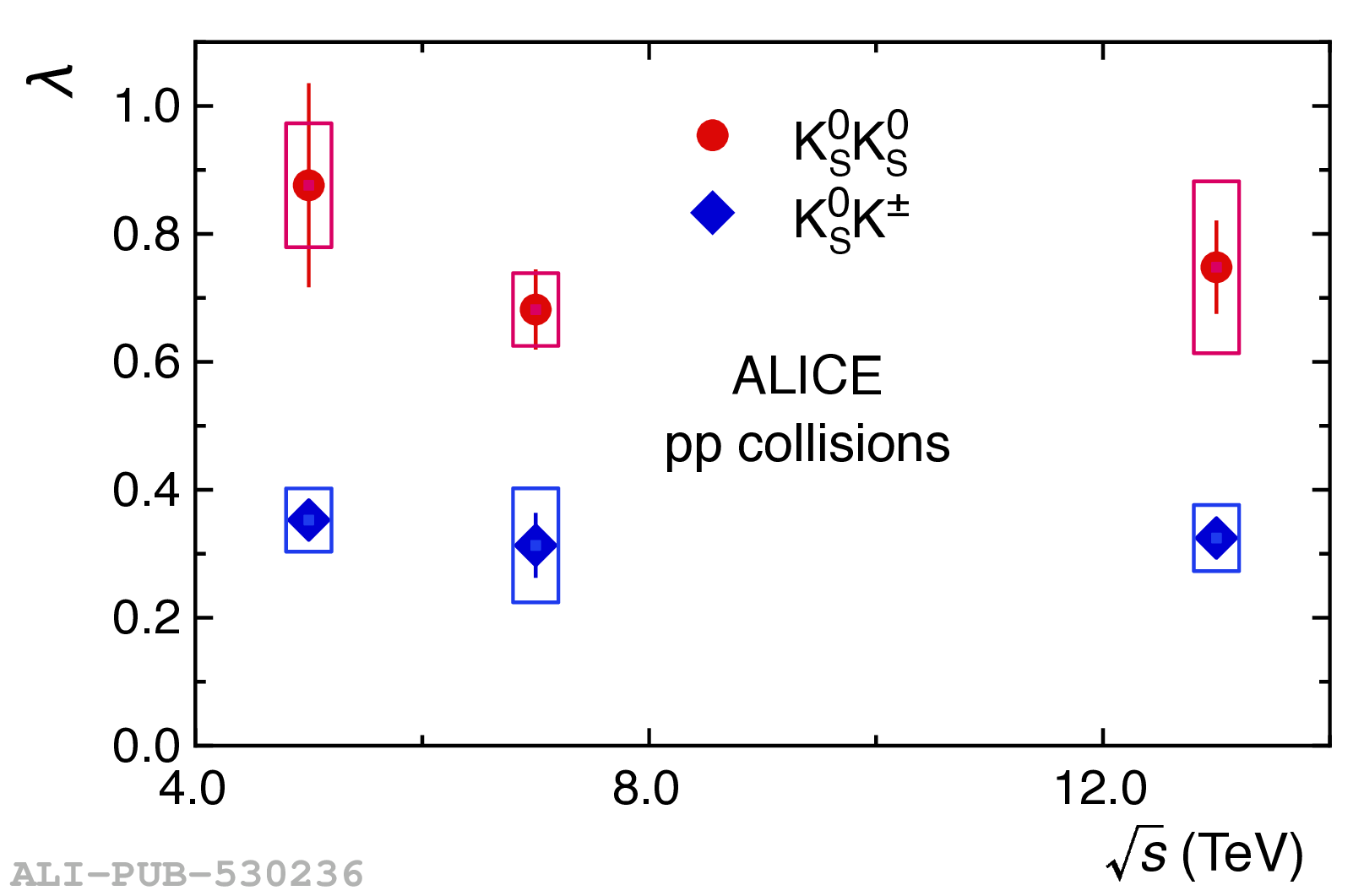
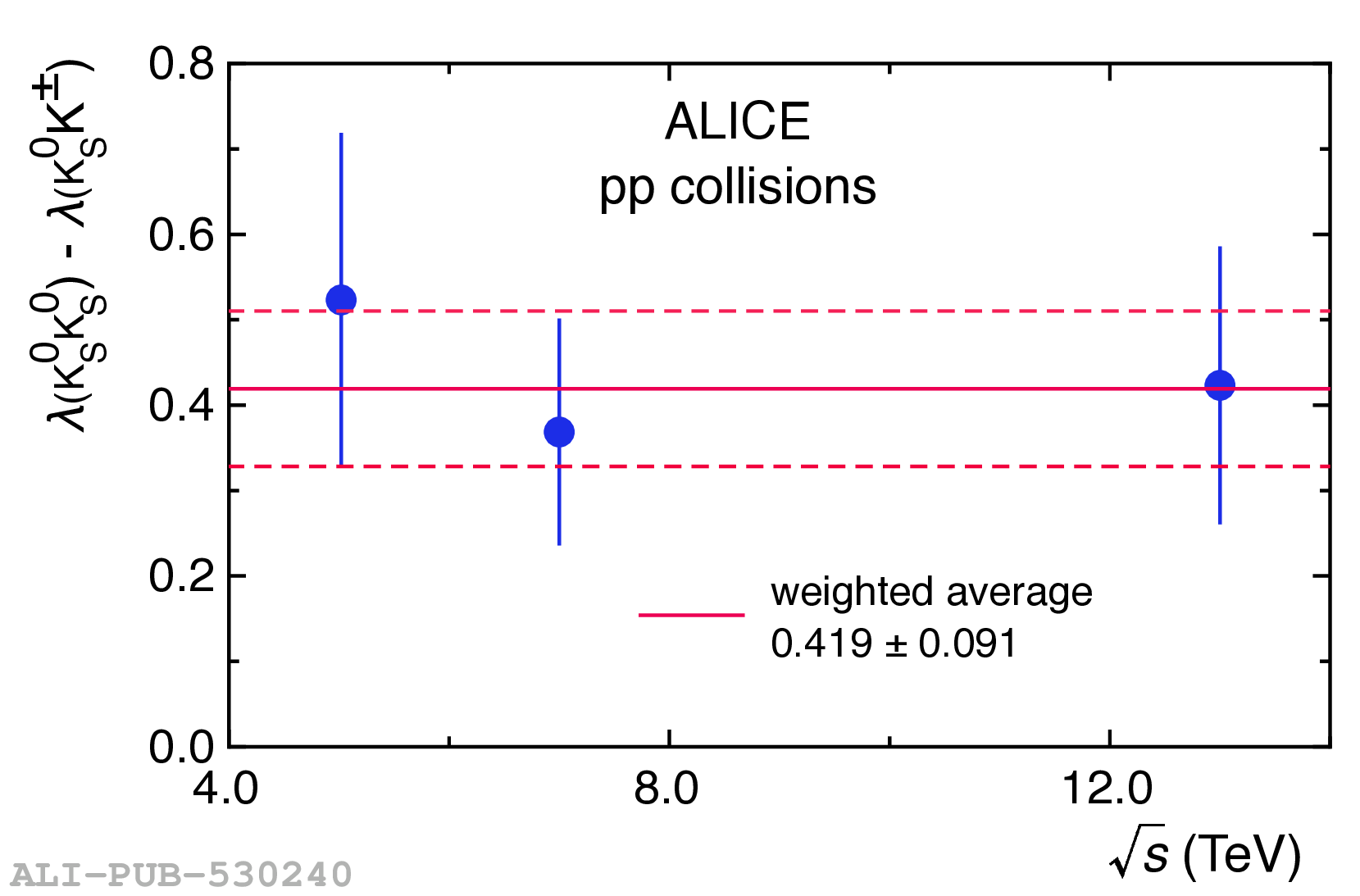
Femtoscopic correlations with the particle pair combinations K$^0_{\rm S}$K$^0_{\rm S}$ and K$^0_{\rm S}$K$^\pm$ are studied in pp collisions at $\sqrt{s}=5.02$ and $13$ TeV by the ALICE experiment. At both energies, boson source parameters are extracted for both pair combinations, by fitting models based on Gaussian size distributions of the sources, to the measured two-particle correlation functions. The interaction model used for the K$^0_{\rm S}$K$^0_{\rm S}$ analysis includes quantum statistics and strong final-state interactions through the $f_0(980)$ and $a_0(980)$ resonances. The model used for the K$^0_{\rm S}$K$^\pm$ analysis includes only the final-state interaction through the $a_0$ resonance. Source parameters extracted in the present work are compared with published values from pp collisions at $\sqrt{s}=$ 7 TeV and the different pair combinations are found to be consistent. From the observation that the strength of the K$^0_{\rm S}$K$^0_{\rm S}$ correlations is significantly greater than the strength of the K$^0_{\rm S}$K$^\pm$ correlations, the new results are compatible with the $a_0$ resonance being a tetraquark state of the form $(q_1,\overline{q_2}, s, \overline{s})$, where $q_1$ and $q_2$ are $u$ or $d$ quarks.
Phys.Lett.B 833 (2022) 137335
e-Print: arXiv:2111.06611 | PDF | inSPIRE
Figure group