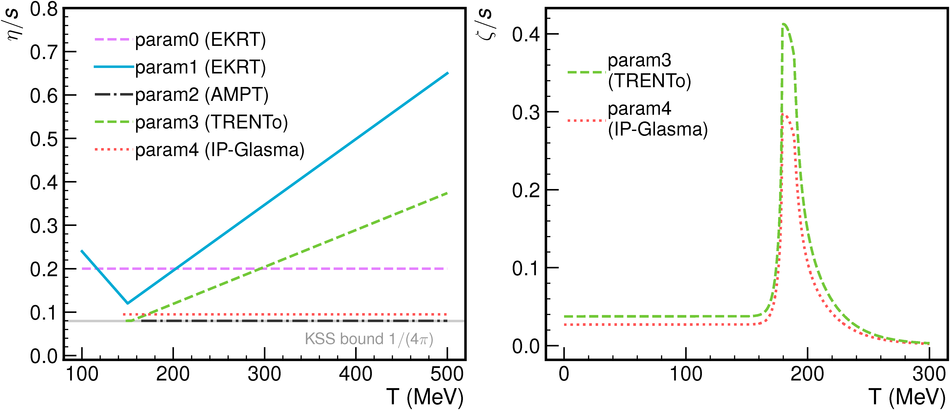
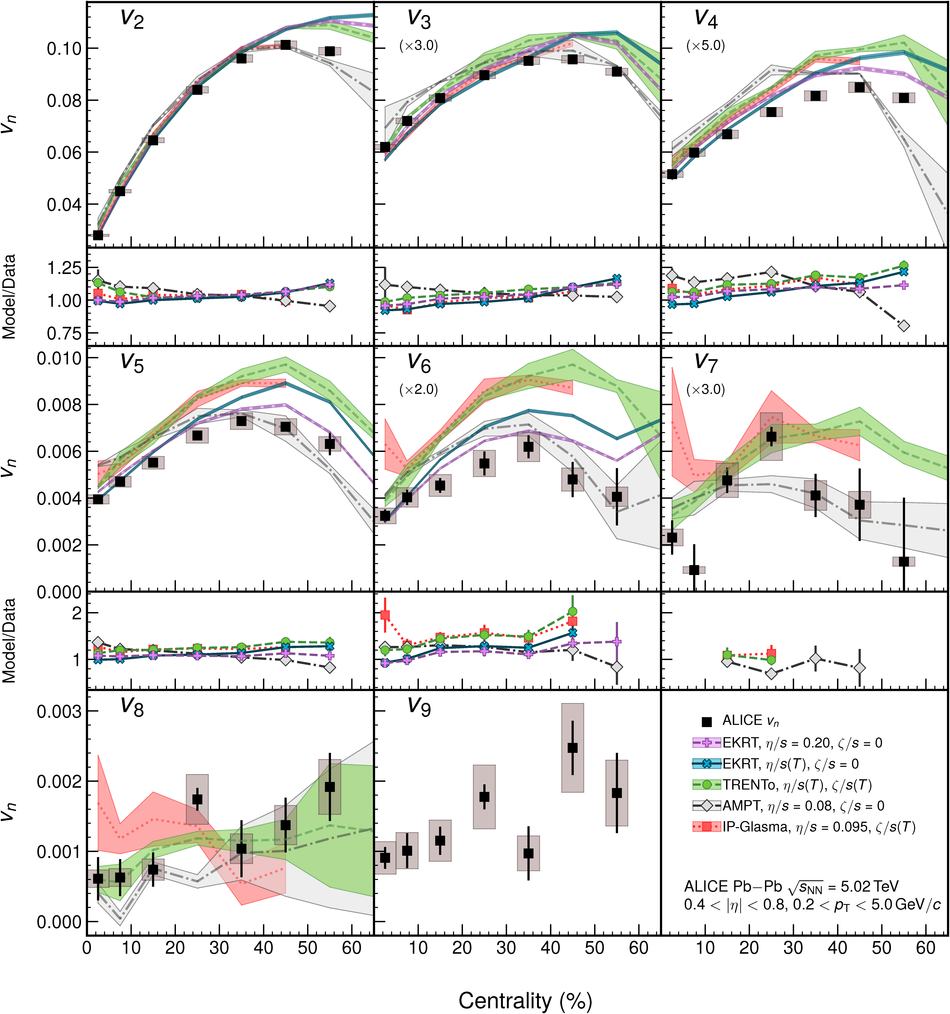
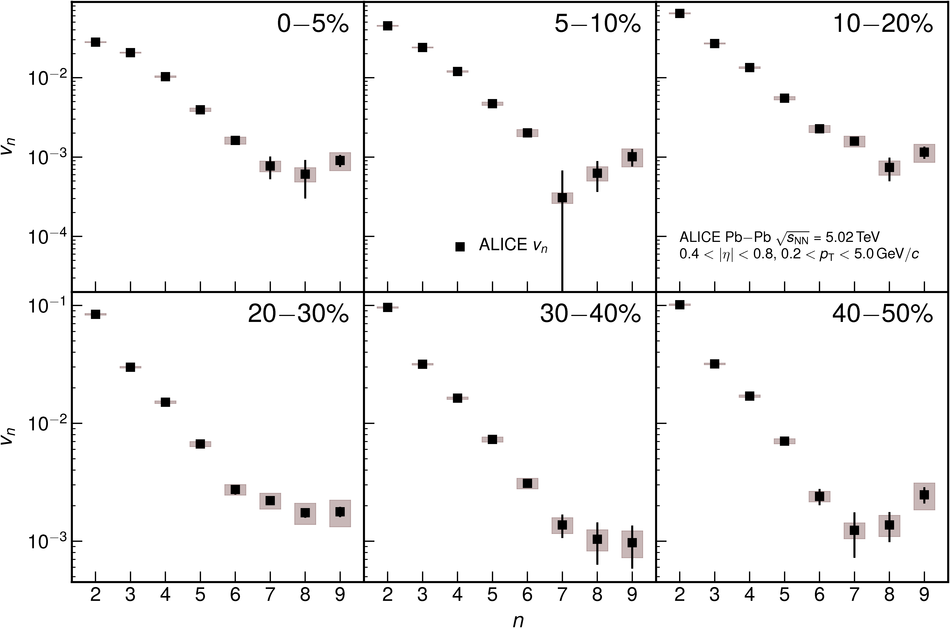
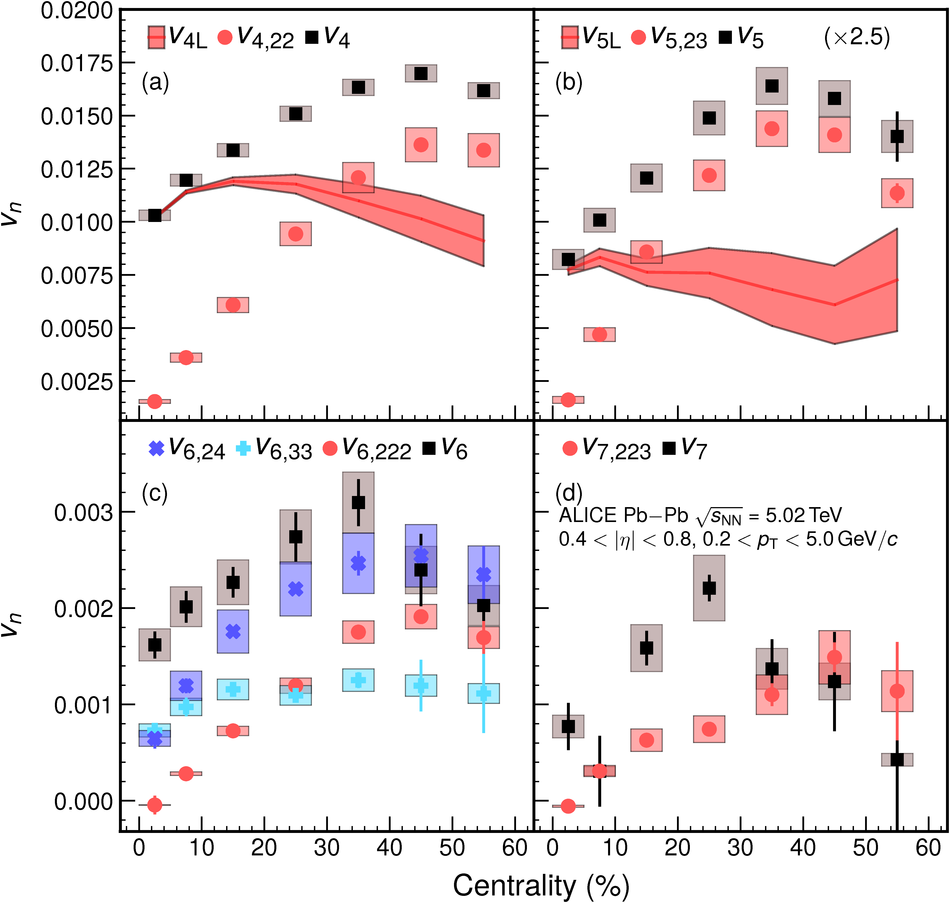
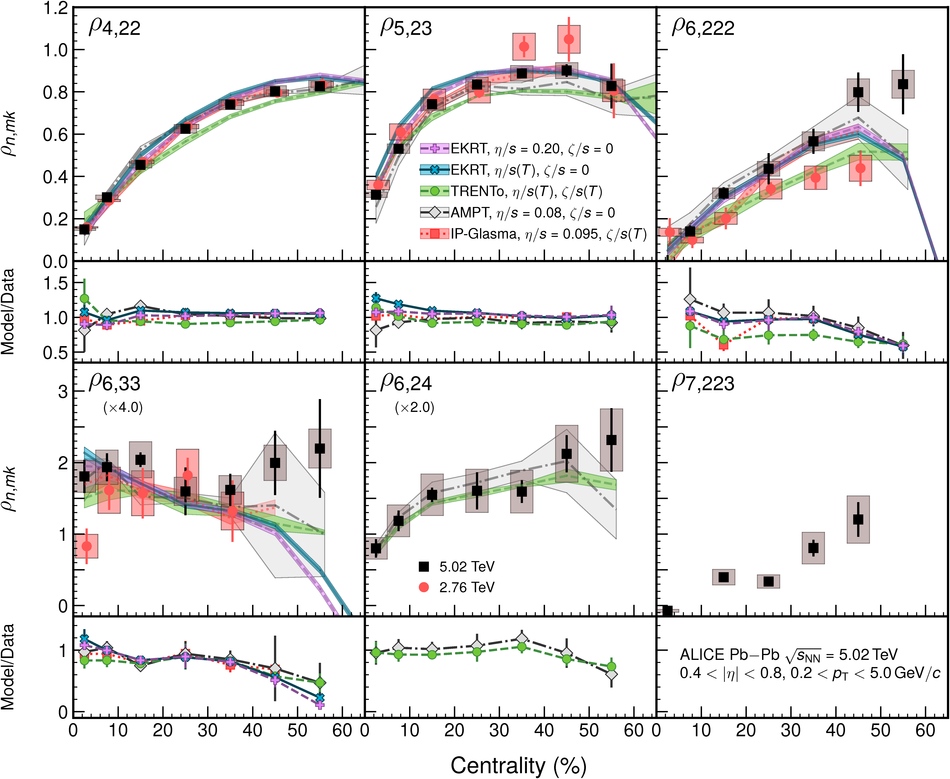
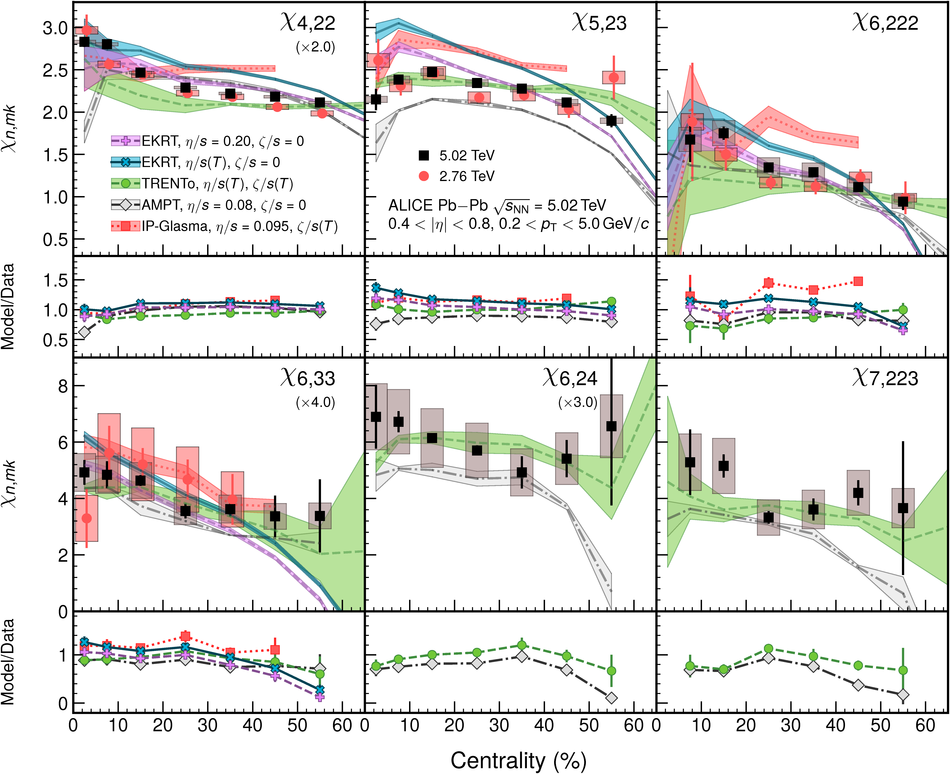
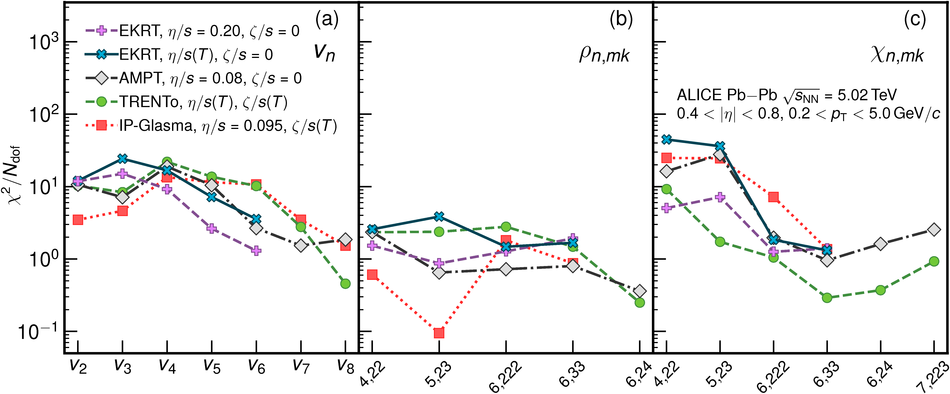
Anisotropic flow coefficients, $v_n$, non-linear flow mode coefficients, $\chi_{n,mk}$, and correlations among different symmetry planes, $\rho_{n,mk}$ are measured in Pb-Pb collisions at $\sqrt{s_\rm{NN}}=5.02$ TeV. Results obtained with multi-particle correlations are reported for the transverse momentum interval $0.2<~p_\rm{T}<~5.0$ GeV/$c$ within the pseudorapidity interval $0.4<~|\eta|<~0.8$ as a function of collision centrality. The $v_n$ coefficients and $\chi_{n,mk}$ and $\rho_{n,mk}$ are presented up to the ninth and seventh harmonic order, respectively. Calculations suggest that the correlations measured in different symmetry planes and the non-linear flow mode coefficients are dependent on the shear and bulk viscosity to entropy ratios of the medium created in heavy-ion collisions. The comparison between these measurements and those at lower energies and calculations from hydrodynamic models places strong constraints on the initial conditions and transport properties of the system.
JHEP05 (2020) 085
HEP Data
e-Print: arXiv:2002.00633 | PDF | inSPIRE
CERN-EP-2019-287