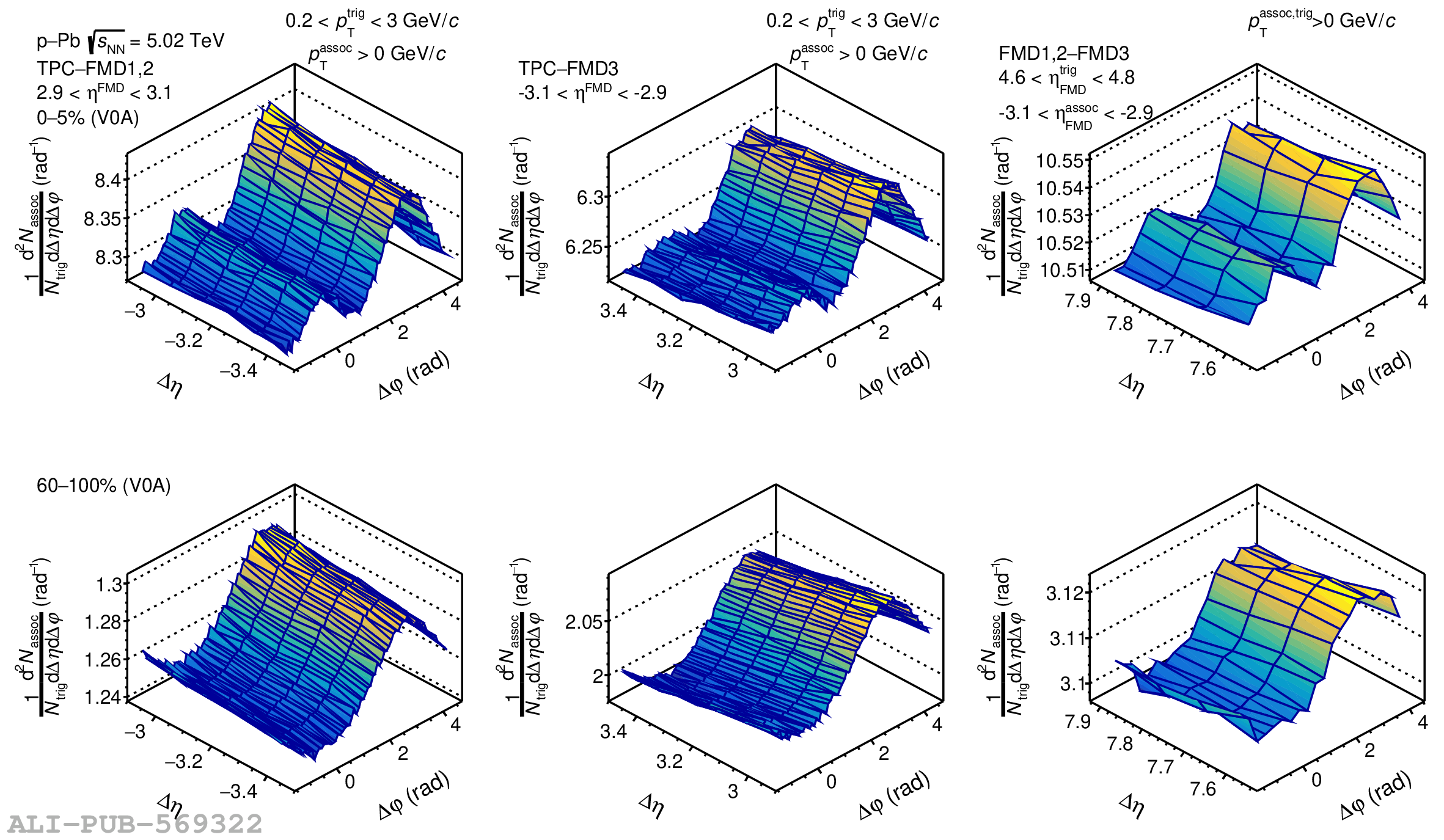
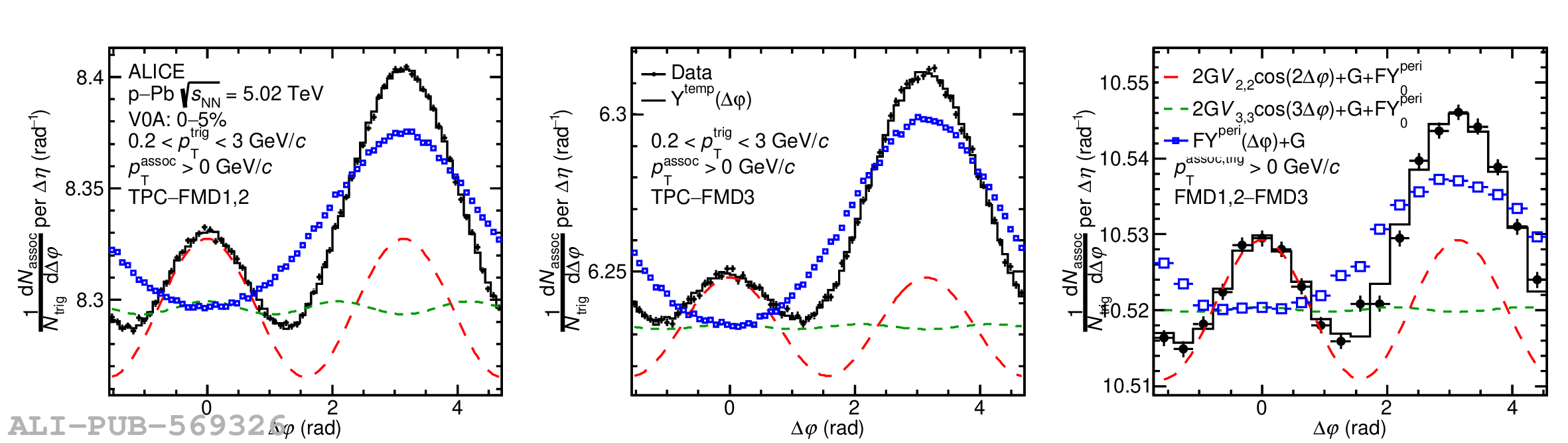
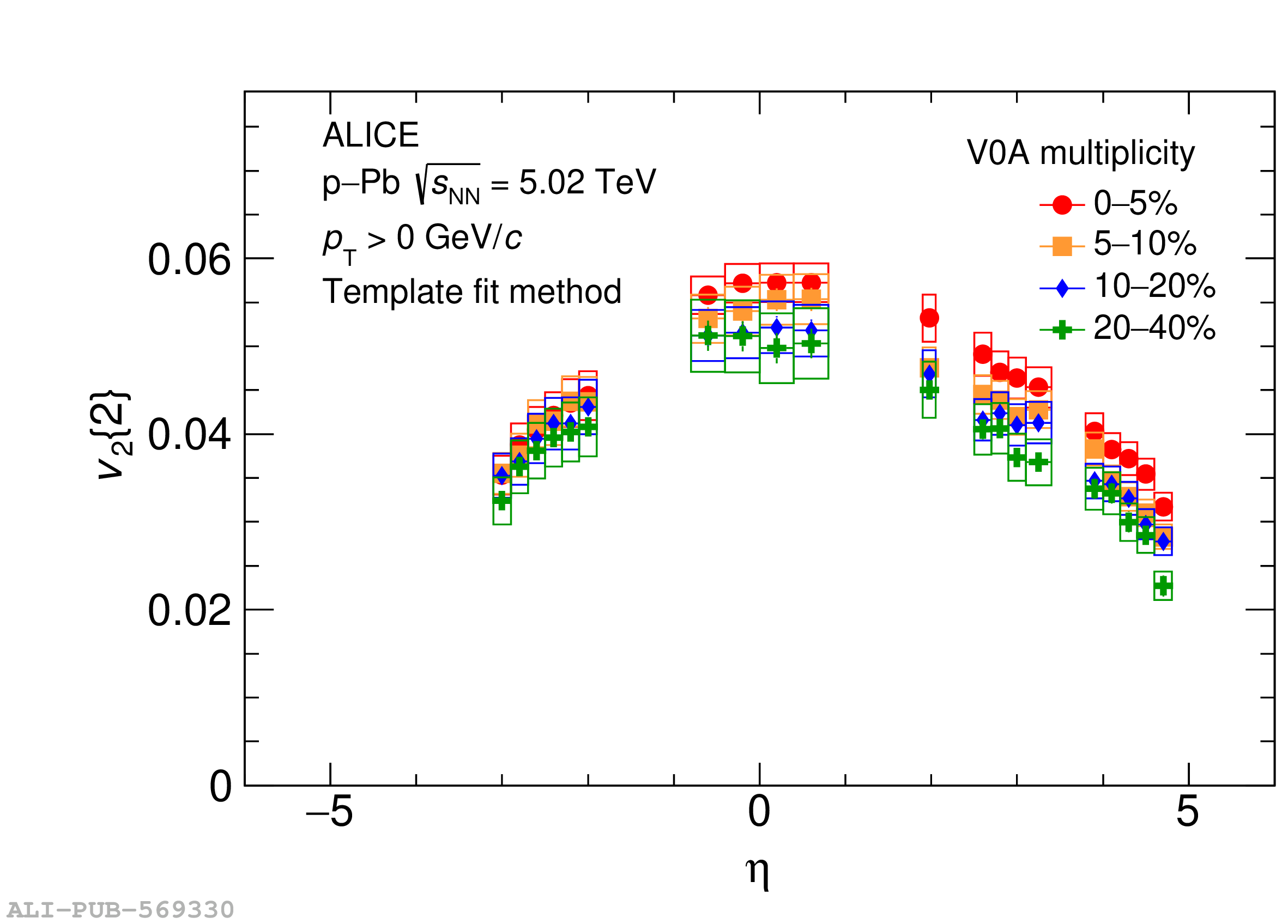
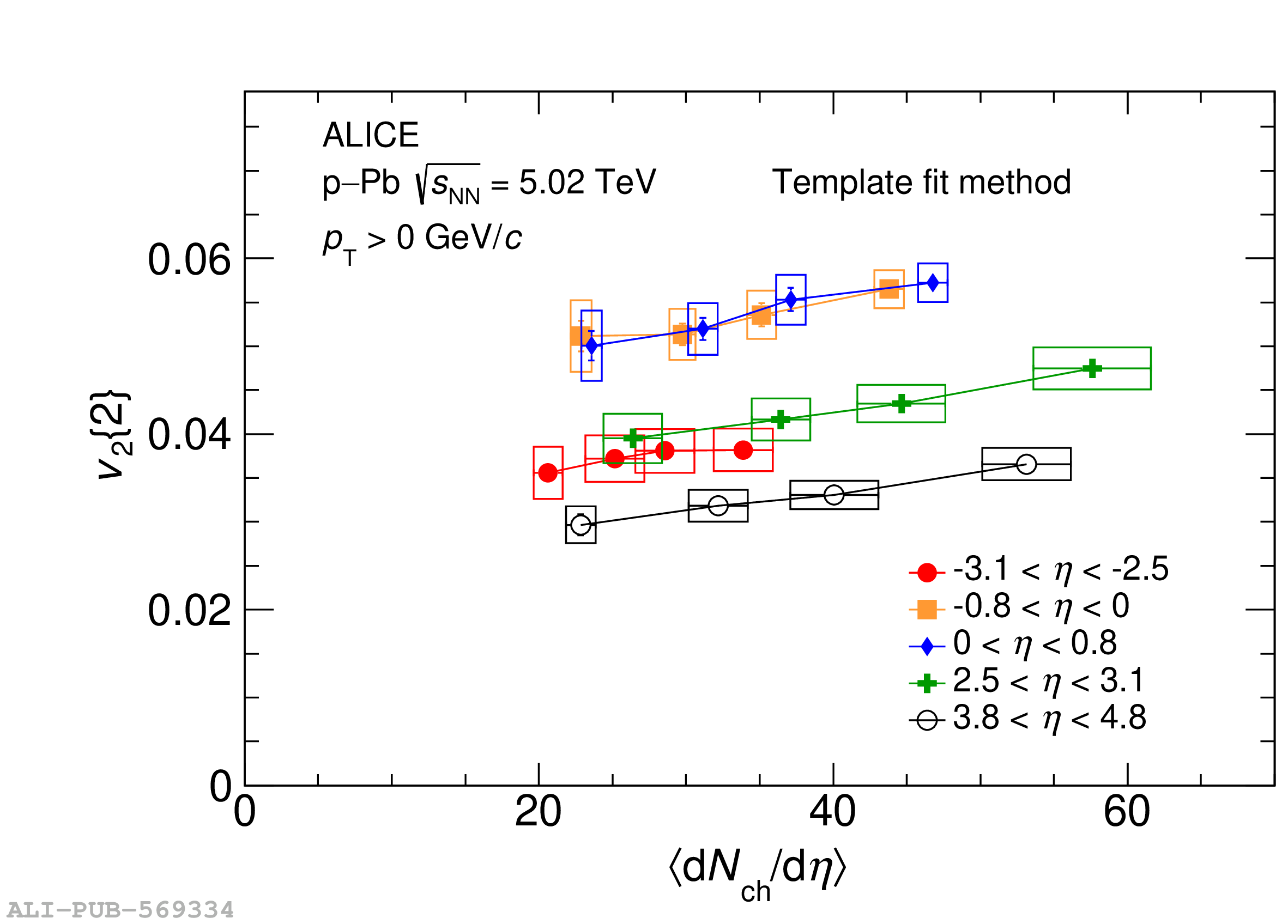
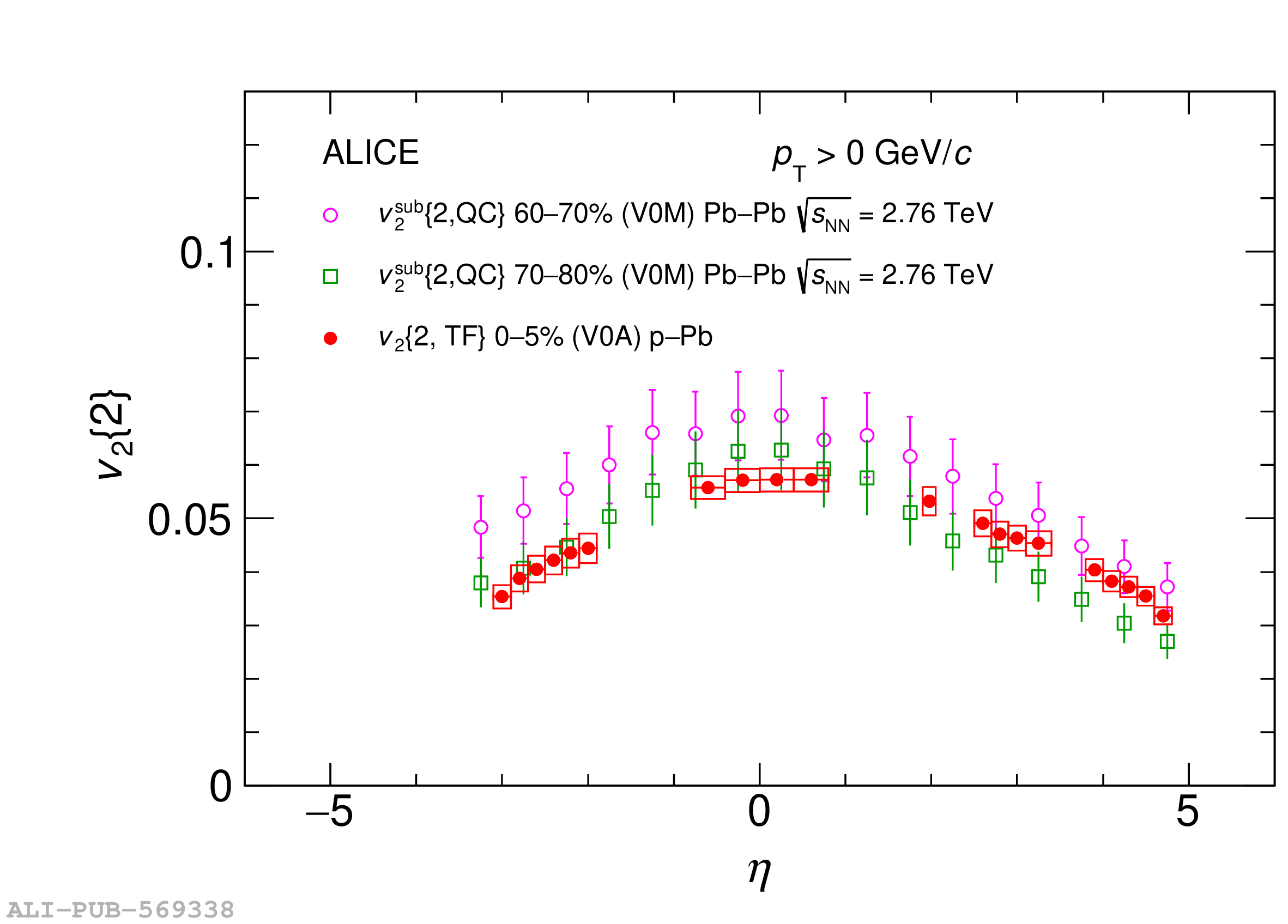
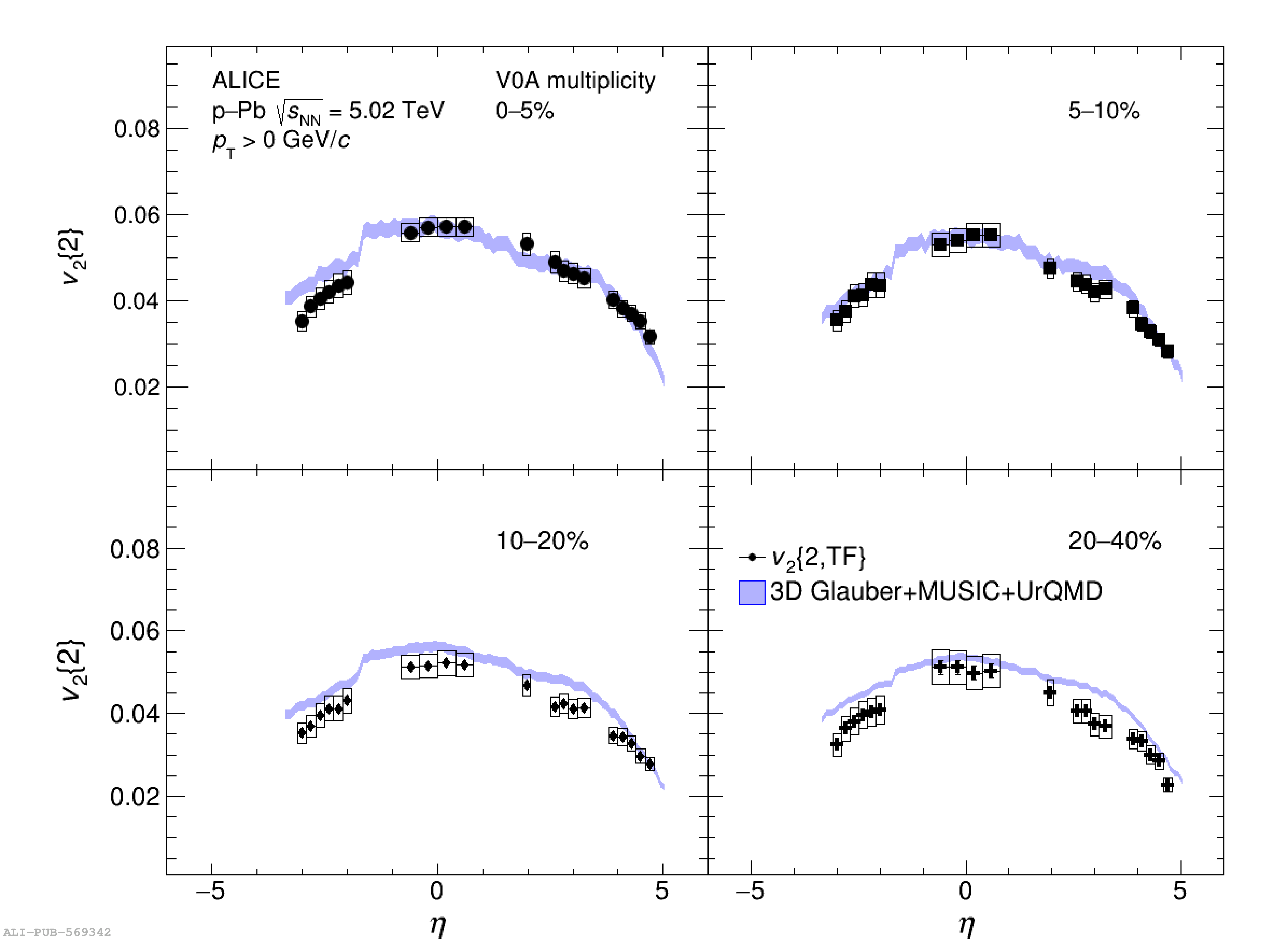
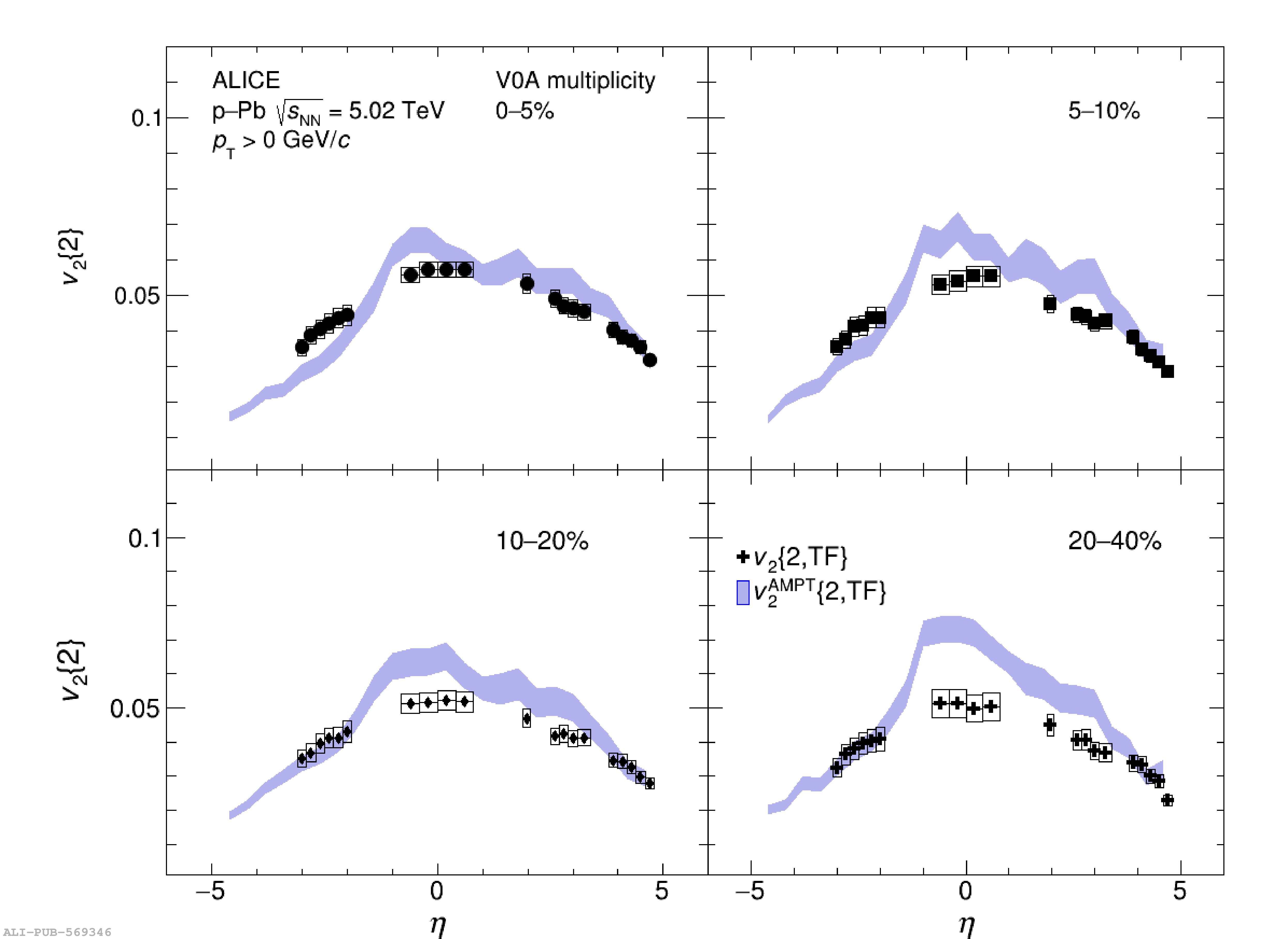
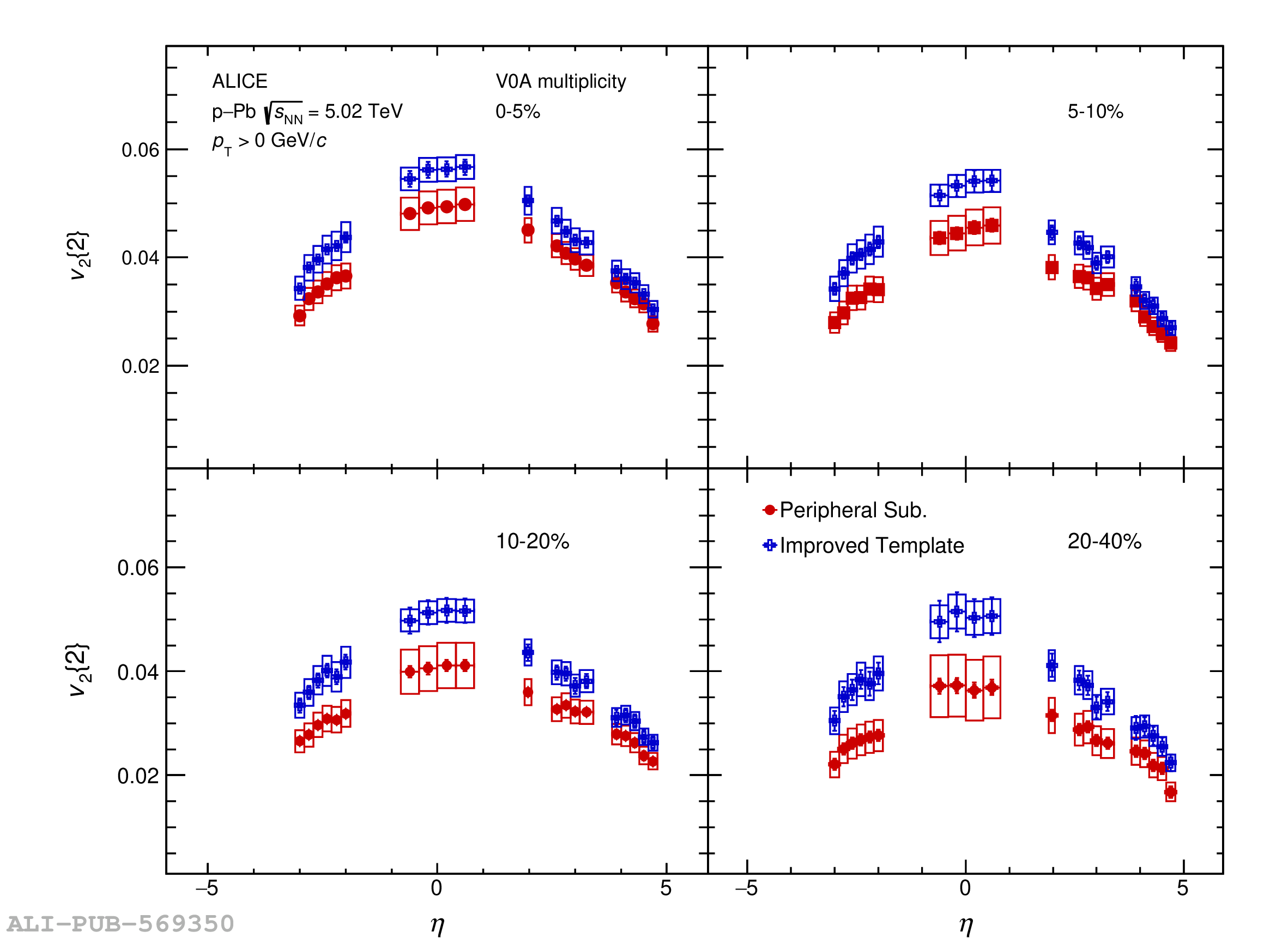
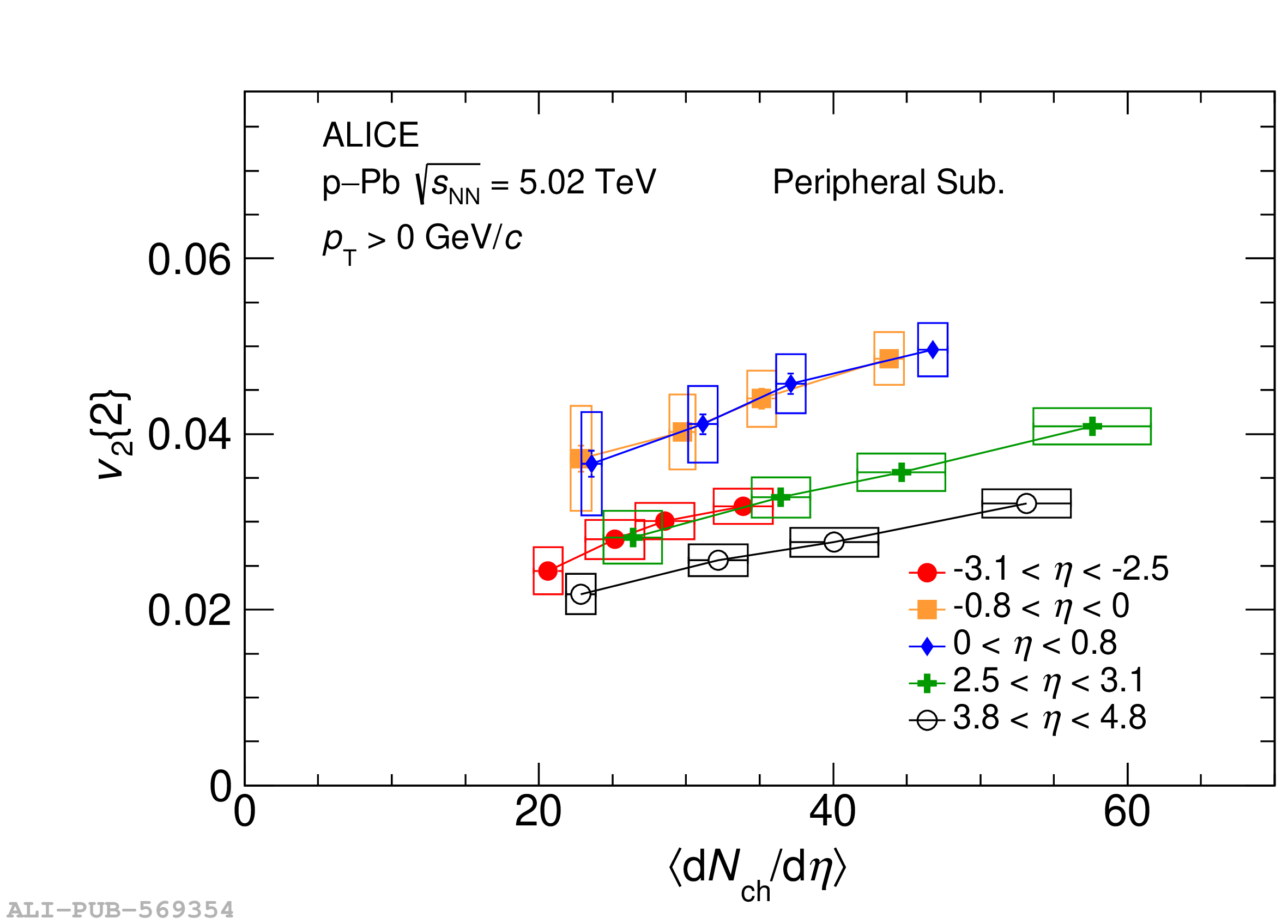
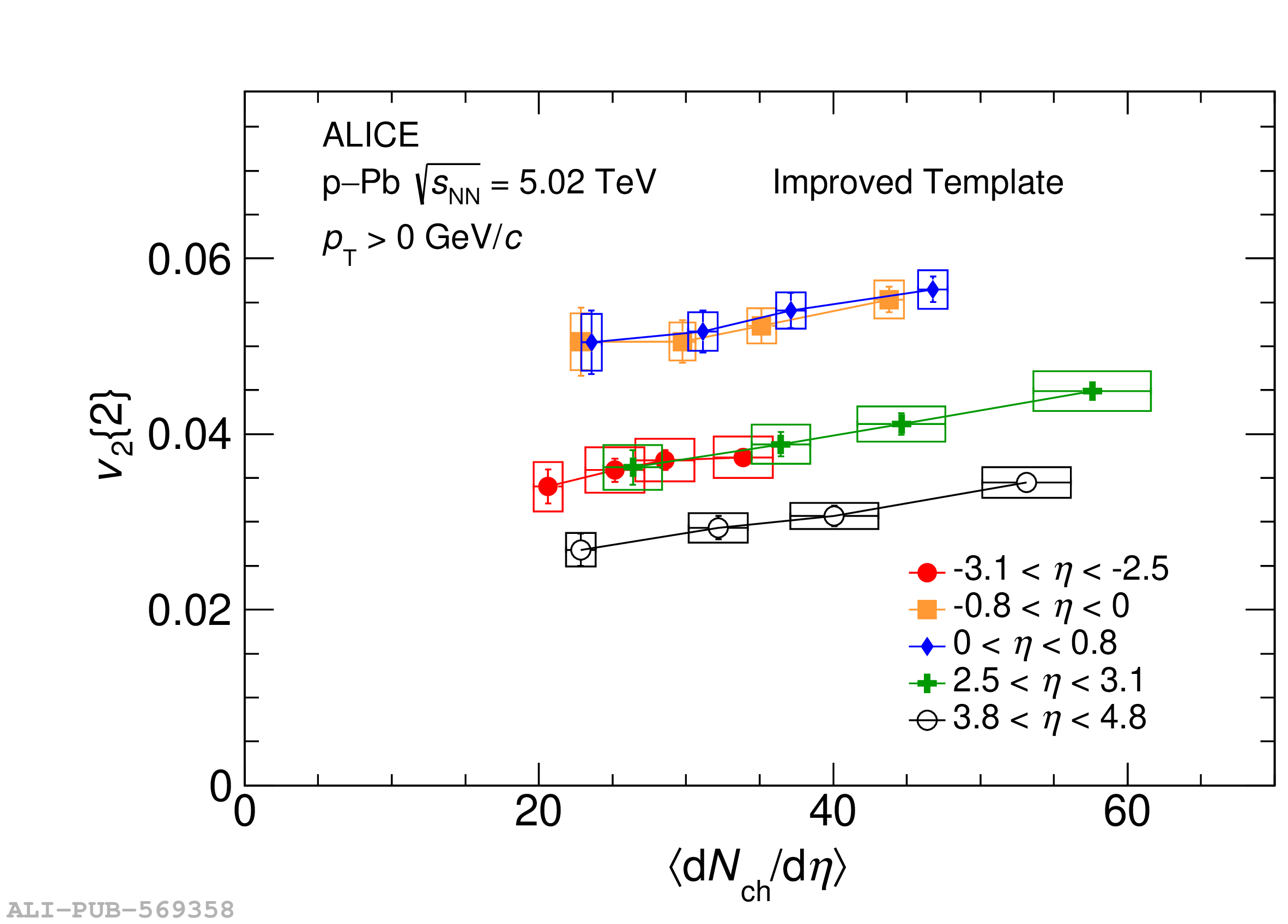
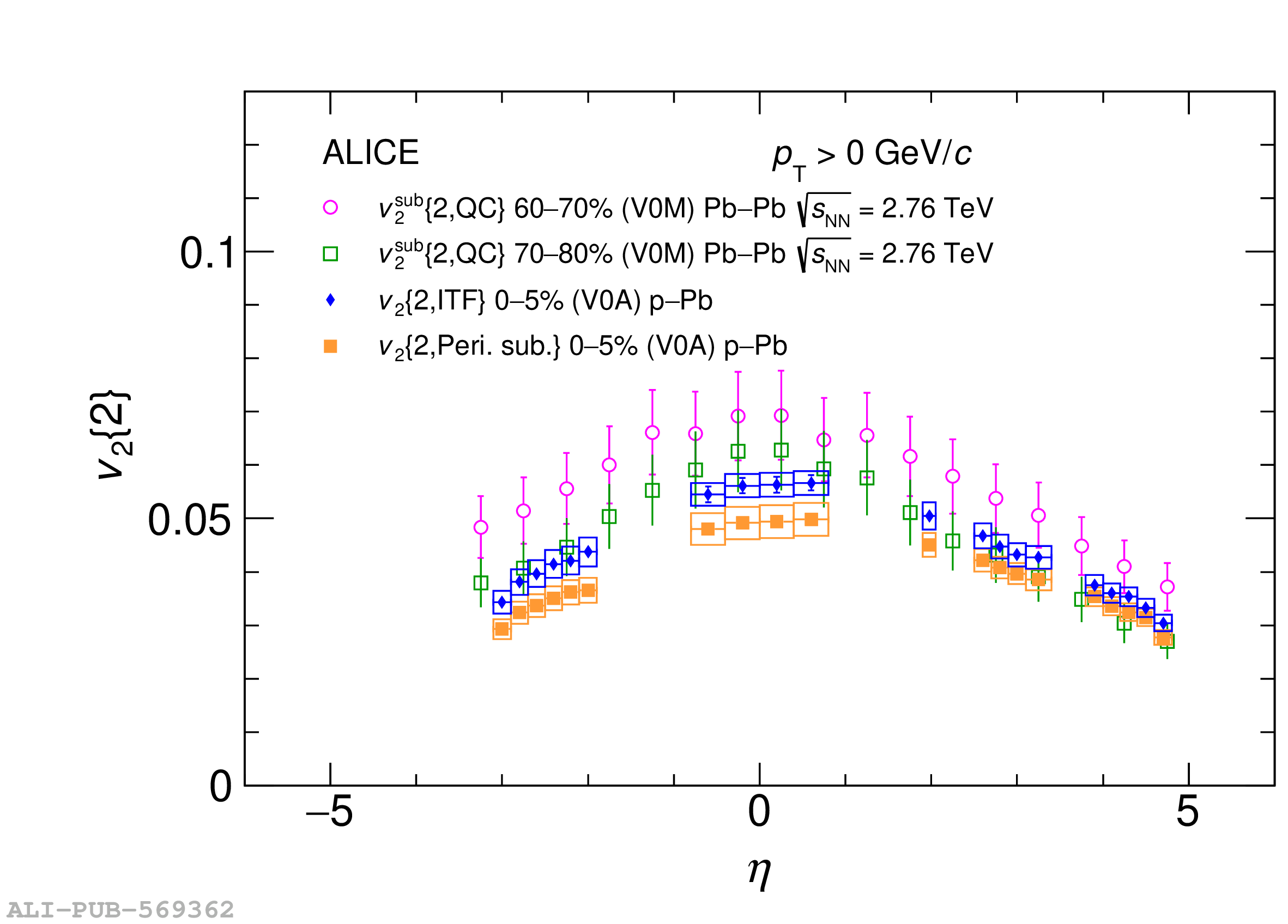
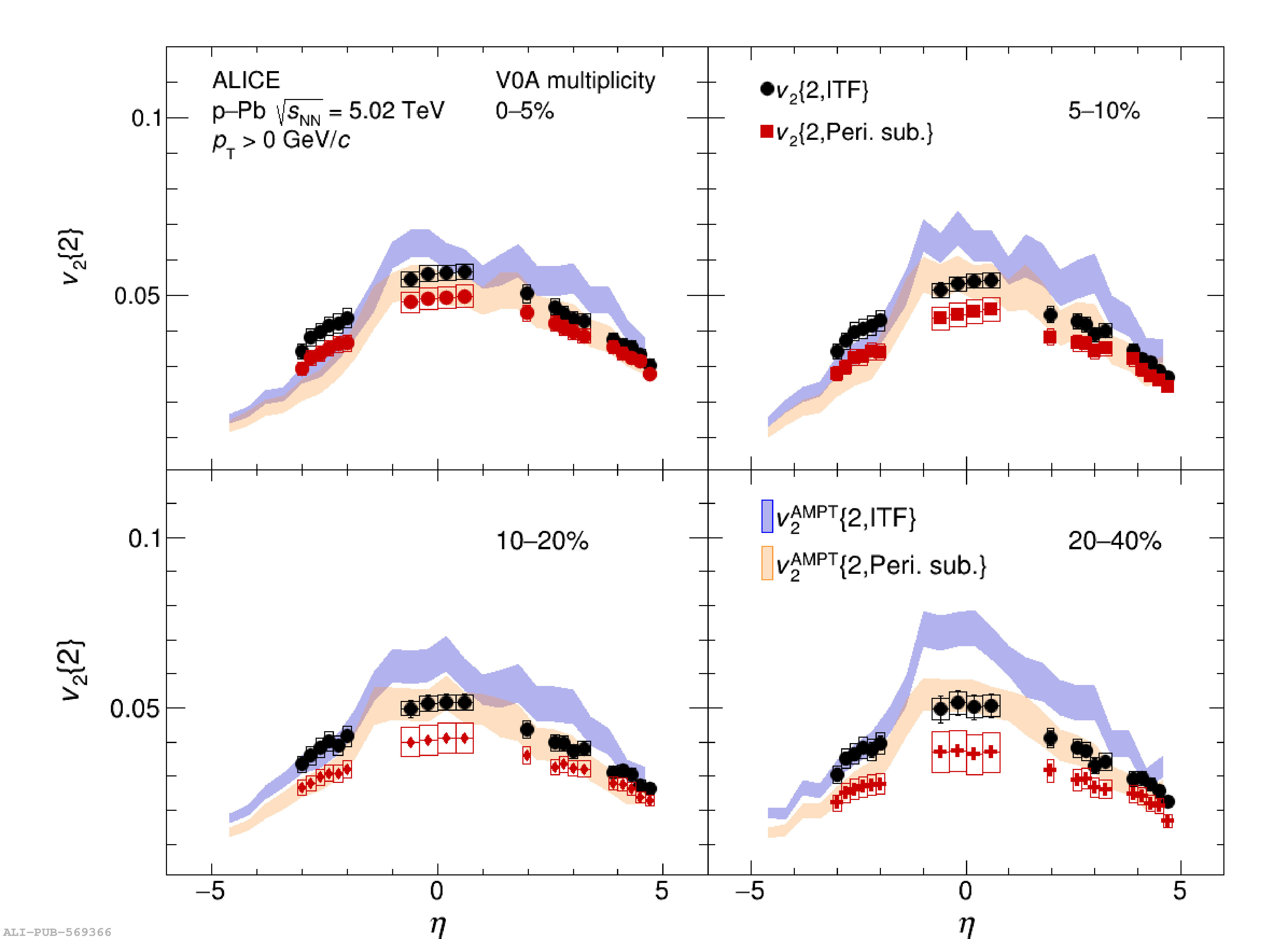
Correlations in azimuthal angle extending over a long range in pseudorapidity between particles, usually called the "ridge" phenomenon, were discovered in heavy-ion collisions, and later found in pp and p$-$Pb collisions. In large systems, they are thought to arise from the expansion (collective flow) of the produced particles. Extending these measurements over a wider range in pseudorapidity and final-state particle multiplicity is important to understand better the origin of these long-range correlations in small-collision systems. In this Letter, measurements of the long-range correlations in p$-$Pb collisions at $\sqrt{s_{\rm NN}} = 5.02$ TeV are extended to a pseudorapidity gap of $\Delta\eta \sim 8$ between particles using the ALICE, forward multiplicity detectors. After suppressing non-flow correlations, e.g., from jet and resonance decays, the ridge structure is observed to persist up to a very large gap of $\Delta\eta \sim 8$ for the first time in p$-$Pb collisions. This shows that the collective flow-like correlations extend over an extensive pseudorapidity range also in small-collision systems such as p$-$Pb collisions. The pseudorapidity dependence of the second-order anisotropic flow coefficient, $v_{2}({\eta})$, is extracted from the long-range correlations. The $v_{2}(\eta)$ results are presented for a wide pseudorapidity range of $-3.1 <~ \eta <~ 4.8$ in various centrality classes in p$-$Pb collisions. To gain a comprehensive understanding of the source of anisotropic flow in small-collision systems, the $v_{2}(\eta)$ measurements are compared to hydrodynamic and transport model calculations. The comparison suggests that the final-state interactions play a dominant role in developing the anisotropic flow in small-collision systems.
JHEP 01 (2024) 199
HEP Data
e-Print: arXiv:2308.16590 | PDF | inSPIRE
CERN-EP-2023-196
Figure group