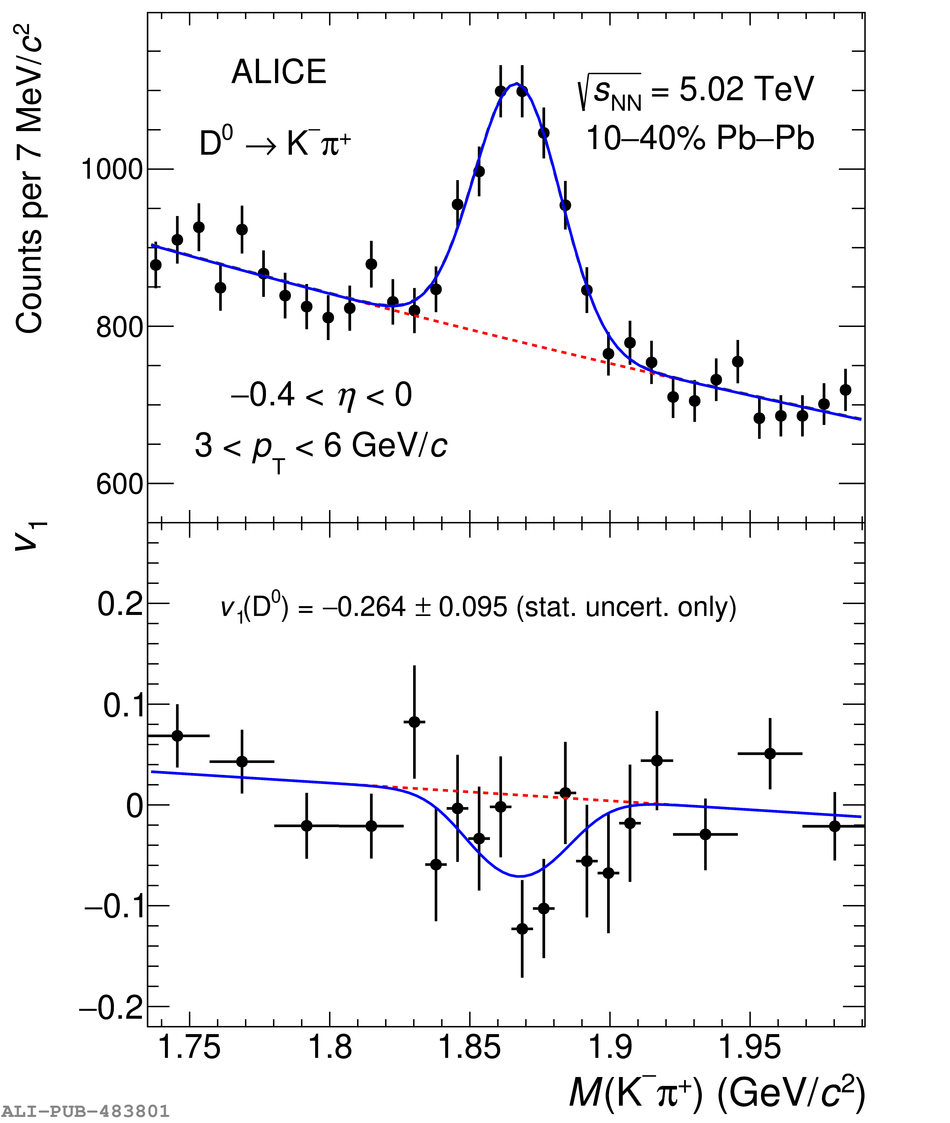
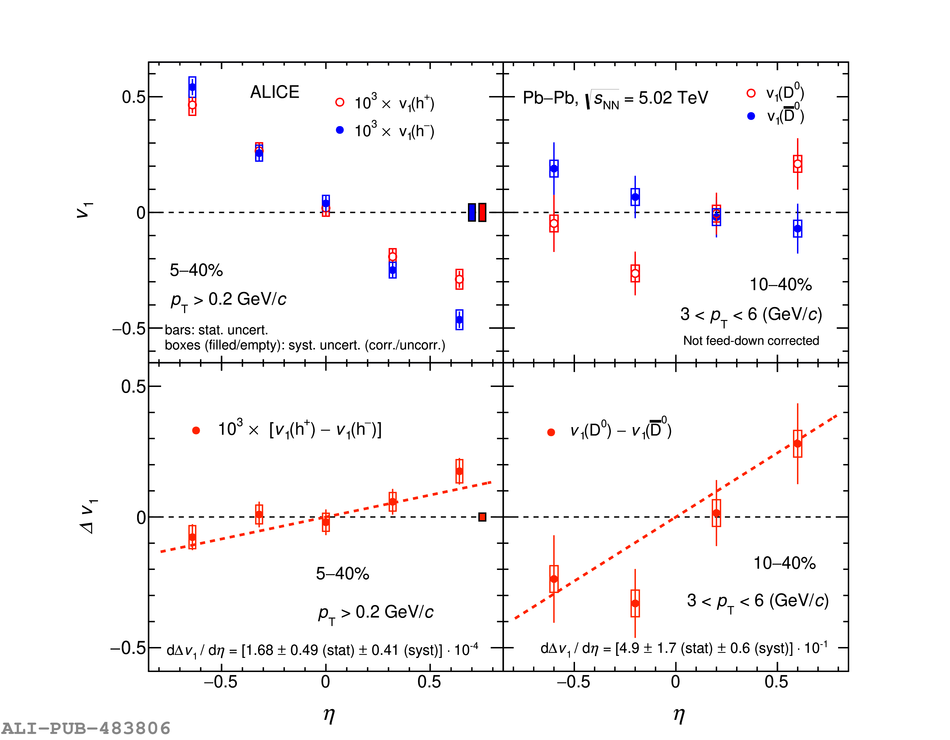
The first measurement at the LHC of charge-dependent directed flow ($v_{1}$) relative to the spectator plane is presented for Pb-Pb collisions at $\sqrt{s_{\rm NN}}$ = 5.02 TeV. Results are reported for charged hadrons and $\rm D^{0}$ mesons for the transverse momentum intervals $p_{\rm T}>0.2$ GeV/$c$ and $3<~p_{\rm T}<~$ 6 GeV/$c$ in the 5-40% and 10-40% centrality classes, respectively. The difference between the positively and negatively charged hadron $v_{1}$ has a positive slope as a function of pseudorapidity $\eta$, ${\rm d}\Delta{v_1}/{\rm d}\eta=$[1.68 $\pm$ 0.49 (stat.) $\pm$ 0.41 (syst.)] $\times 10^{-4}$. The same measurement for $\rm D^{0}$ and $\rm\bar{D}{}^0$ mesons yields a positive value ${\rm d}\Delta{v_1}/{\rm d}\eta$= [4.9 $\pm$ 1.7 (stat.) $\pm$ 0.6 (syst.)]$\times 10^{-1}$, which is about three orders of magnitude larger than the one of the charged hadrons. These measurements can provide new insights into the effects of the strong electromagnetic field and the initial tilt of matter created in non-central heavy-ion collisions on the dynamics of light (u, d, and s) and heavy (c) quarks. The large difference between the observed $\Delta{v_1}$ of charged hadrons and $\rm D^{0}$ mesons may reflect different sensitivity of the charm and light quarks to the early time dynamics of a heavy-ion collision. These observations challenge some of the recent theoretical calculations, which predicted a negative and an order of magnitude smaller value of ${\rm d}\Delta{v_1}/{\rm d}\eta$ for both light-flavour and charmed hadrons.
Phys. Rev. Lett. 125, 022301
HEP Data
e-Print: arXiv:1910.14406 | PDF | inSPIRE
CERN-EP-2019-241